Energy-Efficient Operation of Water Systems through Optimization of Load Power Reduction in Electricity Markets

Chouaïb Mkireb＊ | Abel Dembele | Antoine Jouglet | Thierry Denoeux

Abstract—Demand response (DR) is gaining more and more importance in the architecture of power systems in a context of flexible loads and high share of intermittent generation.Changes in electricity markets regulation in several countries have recently enabled an effective integration of DR mechanisms in power systems.Through its flexible components (pumps, tanks), drinking water systems are suitable candidates for energy-efficient DR mechanisms.However, these systems are often managed independently of power system operation for both economic and operational reasons.Indeed, a sufficient level of economic viability and water demands risk management are necessary for water utilities to integrate their flexibilities to power system operation.In this paper,we proposed a mathematical model for optimizing pump schedules in water systems while trading DR blocs in a spot power market during peak times.Uncertainties about water demands were considered in the mathematical model allowing to propose power reductions covering the potential risk of real-time water demand forecasting inaccuracy.Numerical results were discussed on a real water system in France, demonstrating both economic and ecological benefits.

1. lntroduction

The objectives of energy transition involve major changes in the operating mode of transmission and distribution power networks.On the supply side, the world is experiencing a massive integration of renewable decentralized generation.On the demand side, the world is experiencing a rapid increase in electricity consumption[1], mainly due to the development of new usages of electricity: Electric vehicles, heat pumps, etc.Due to the low storage capacity of electricity, these changes make the exercise of balancing the electric power system very difficult, and imply a growing need to develop efficient methods for demand side management[2].Demand management, also known as demand response (DR), is the change in the power consumption of an electric utility consumer in response to a given signal.It requires active consumers in energy markets, known as “prosumers”, adapting their electricity demands to the available generation and price signals in wholesale markets.Industrial processes with storage units (warehouse, electric batteries) are believed to be the best candidates for DR since they have an electric flexibility they can use to optimize their productivity while helping to manage several situations for power network management[3].The benefits from this participation include:

1) Reducing the risk of service interruptions caused by supply shortages, transmissions congestions, or rolling blackouts;

2) Reducing the use of fossil generation units to deal with peak demands and then reducing CO2emissions.

In this paper, the case of a highly energy intensive industry is discussed, which is the drinking water industry.First, the water systems’ flexibility and their potential to reduce peak load are discussed.After, the French model making it possible to trade DR directly to spot markets is presented.Section 3 presents the mathematical model allowing water systems to optimize their participations in the spot market while anticipating uncertainties on water demands.Finally, some numerical results, interpretations, and future directions are discussed.

2. Drinking Water Systems Acting Like Prosumers

This section presents the potential for the electrical flexibility of drinking water systems.Then, the particular DR mechanism considered for our study is discussed.

2.1. Drinking Water Systems Flexibility

Drinking water systems can account for up to 5% of a city’s total electricity consumption[4]and more than two thirds is used by electric pumps[5].In fact, pumps are highly energy-intensive since they operate continuously to ensure a sufficient level of water autonomy to tanks and reservoirs in anticipation of uncertainties on water consumption.At the same time, drinking water systems have a considerable electrical flexibility thanks to the presence of storage units (reservoirs, tanks) and variable speed pumps.Indeed, this flexibility is generally used to optimize energy costs by optimizing the pump schedules according to the different electricity time of use tariffs.

In the past years several advances in smart grid technologies have been achieved and we have seen a progressive change in electricity market rules by regulatory agencies[6].These changes have contributed to the implementation of smart technologies like advanced metering infrastructures and the removal of barriers for DR participation in electricity markets.At the same time, the water industry benefits from the development of both sophisticated supervisory control and data acquisition (SCADA) systems and programmable logic controller (PLC), allowing water utilities to control and optimize the water production process from its capture to final distribution.Thanks to these progresses, drinking water systems could use their flexibilities (through storage units and variable-speed pumps) to improve the power system’s reliability through DR[7].They could act like active prosumers, interact in real time with energy markets and transmission system operators (TSO), and participate in efficient DR programs, by adapting their electricity consumption to the need of the electric power system.

2.2. Demand Response in French Electricity Markets

In France, DR operators are in competition with energy suppliers to value the flexibilities of consumers.They can therefore trade DR on electricity markets without prior agreement of suppliers[8].This opportunity offered to encourage DR operators had been accompanied by important regulatory work to define the rules and modalities for the exchange of financial and energy flows between different market players.

In 2014, France set up a mechanism, called the“NEBEF” mechanism, allowing one to trade DR directly in spot (D–1) power markets as a resource[9].In this context, the DR operator sells on day D–1 at midday, the electricity which will not be consumed on day D by the consumer, and compensates financially the supplier of the site participating in the NEBEF mechanism (see Fig.1).In other terms, the DR operator buys the energy from the supplier at a regulated price, called compensation, to compensate him for the energy he has injected into the network.The supplier continues then its injections as planned and the DR operator sells in the spot market the energy that the consumer will not have to consume.

France has implemented this mechanism to reduce peak power load, especially during cold winters.These periods experience load growth of 2300 MW between 18:00 to 20:00 for each degree Celsius less of temperature,in what we call a thermo-sensibility phenomenon[10].The mechanism contributes to the reduction of the use of fossil power plants, and hence CO2emissions, to deal with these peak load periods.In the absence of dynamic energy pricing in French retail markets, this mechanism is also a way to expose end-consumers to dynamic electricity prices by encouraging them to modulate their consumption according to wholesale market price signals.

For the NEBEF mechanism, each DR bid on the spot market must constitute at least 100 kW of power reduction.In addition, DR bids cannot exceed a maximum of two hours per block[9].The method of estimating the real load curtailed by the DR operator during a DR event consists in comparing two curves:

1) Reference curve: The minimum between the mean electric loads just before (past reference) and just after(post reference) the DR event, over a period of time equal to that of the DR event.

2) DR curve: Mean electric load during the DR event.

The load curtailed during a DR event is equal to the difference between the reference curve and the DR curve (see Fig.2).This estimation method is called the corrected double reference method and is used by the French transmission system operator RTE(Réseau Transport d’Electricité) in order to quantify the real power reduction achieved by the consumer.

Compensation prices are regulated and fixed at the end of each year by RTE on the basis of observed spot prices during the year[9].They depend on the season,type of the day (working/non-working), and time(peak/off-peak hours).

Fig.1.NEBEF mechanism.

Fig.2.Illustration of reference periods and load curtailment.

3. Mathematical Model

The optimal pump scheduling problem in drinking water systems is a long-standing problem in [11].It is an NPhard problem[12]aiming to find optimal schedules of pumps while meeting all water system constraints at minimum cost.Our study proposes to integrate another economic stake in the objective function, which is the revenue earned by trading DR on the spot market through the NEBEF mechanism.

The hypothesis made in this study is that the water utility acts on the spot market as a DR operator and seeks to maximize its own profits.Both the biddings of the water utility on the power exchange and the operation of pumps are scheduled one day ahead in order to maximize the overall profitability of the system while respecting various constraints of the water network.DR blocs are put on sale in winter and only during the evening peak(18:00 to 20:00) since it corresponds to annual national peak load, where the power system needs DR to replace the high-cost high-emissions peak generation units (coal, gas, and diesel).

However, planning one day ahead (day D–1) the amount of electricity consumption to be reduced (in day D)during the peak times represents a challenge for water utilities due to the uncertainties about water demands over the network.It requires well-formulated operating schedules for pumps and risk-management to ensure that the water level in tanks remains in the operational range at minimum cost.In addition, a minimum of financial viability is required from water utilities to participate in DR schemes.A key factor to address these challenges is the use of mathematical programming to produce optimal pump operation schedules with respect to all water network constraints while maximizing the utility of DR in markets.

The objective is to find, one day ahead, optimal decisions on the functioning of equipment and the DR power reduction to sell on the market, while meeting all the constraints of the water system and covering risks linked to uncertainties of the water demand.For the modeling of uncertainties, we consider that we have an initial forecast of the water demand, but it is uncertain and assumed to be included in an interval.Step-times are discretized into one-hour interval periods.The following notations are then used:

·st: Tanklevel at periodt;

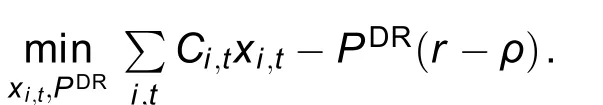
The decision variables are the state of pumps and the power to put on sale for DR.The first term of the objective function is related to energy costs and the second term is linked to DR financial benefits.

In general, the constraints related to the management of a water system include physical constraints (minimum and maximum operating levels of reservoirs), regulatory constraints (conditions of water resources withdrawal imposed by public authorities), and operational constraints (specific management modes related to each system).Since all these constraints are often encountered in the literature on drinking water systems, we will refer to them as the drinking water systems classical constraints, to which the following equations are added:

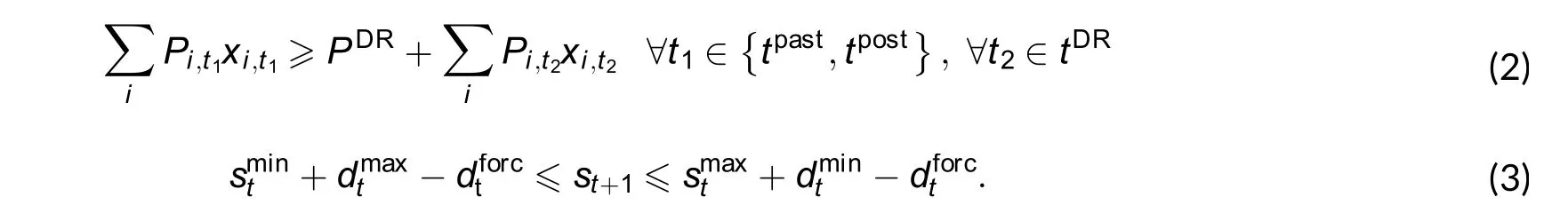
Equation (1) represents the minimum power reduction in kW that can be traded for the NEBEF mechanism.Equation (2) models the past and post reference powers before and after the DR event.Finally, constraint (3)forces the level of the reservoir for the next time step to be in the operational-management field of the reservoir(between minimum and maximum volumes), corrected with the difference between the forecasted and extreme demands.

The final problem of optimizing DR bids and the operation of pumps on day ahead can be written as a combination of the objective function, drinking water systems classical constraints, and constraints (1) to (3).The problem is formulated as the following mixed integer linear programming (MILP) problem:

Problem 1:

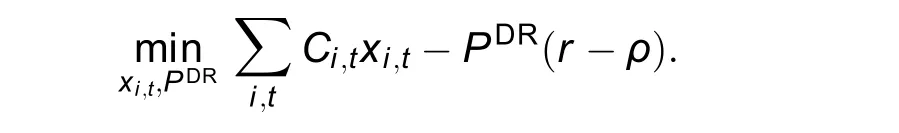
Subject to:

·Drinking water systems classical constraints;

·Constraints (1) to (3).

4. Numerical Results

In this section, optimal water system management with DR participation is evaluated.Then, water system strategies regarding bids on the spot market are analyzed, according to spot price scenarios.Finally, the benefits for using the flexibility of the water system for power system management are discussed.Optimization Problem 1 described in Section 3 has been resolved for a range of market price scenarios using the branch and bound algorithm (B&B) under the CPLEX optimization solver[13].

4.1. Simulation Data

A real drinking water system in France was used as benchmark.This system contains one production plant, 11 pumping stations, and 14 distribution reservoirs.The average daily water demand of the system is about 50000 m3in winter.

A water demand history of 32 scenarios is available in winter for the system.These scenarios were used to build the forecasted maximum and minimum hourly water demand profiles.Extreme demands (max and min) were constructed by taking upper and lower envelopes over a proportion p of historic scenarios.The choice of the scenarios on which the envelopes were calculated is such that the area between maximum and minimum envelopes is minimal (the mathematical method for extracting these scenarios is not detailed in this article).

As shown in Fig.3, the hourly water demand profile is similar to that of the electricity load.The forecasted profile was built by taking an arithmetic mean on the 32 historical demands.Extreme demands were built by taking upper and lower bound on a fixed number of 25 scenarios, corresponding to 80% of scenarios.The 80% value was chosen because it corresponds to a safe level of control of uncertainties.

For numerical simulations, we used data for the year 2016 in winter, corresponding to spot and compensation prices between 18:00 to 20:00.Spot prices are available in the French power exchange (Epex Spot) website.The compensation price was 56.10 €/MWh in winter 2016 at peak times[9].

4.2. Optimal Water System Management

Resolution of Problem 1 yields optimal schedules for pumps, an optimized management of tank levels covering potential water demand uncertainties, and optimal peak power reduction to be sold on the market via the DR NEBEF mechanism.For an average spot price of 81 €/MWh for the year 2016, some simulation results are presented in Figs.4 and 5.

As shown in Fig.4, pumping operations are minimized for the water system during peak hours(06:00 to 20:00) to meet the demand at minimum cost.Meanwhile, tank levels gradually decrease, without reaching the minimum level of security in order to anticipate possible water demand forecasting errors(see Fig.5).However, a higher activity of pumps is observed at off-peak hours (20:00 to 06:00) to take advantage of the cheapest electricity tariffs.During the DR period, the tanks level drop as pumping operations isminimized, but it does not reach the minimum level of security in anticipation of unexpected water demand hazards.On the other hand, some peak hours experience pumping operations:

1) During the morning water peak period 08:00 when the water demand is very high;

2) Midday at 13:00 to anticipate possible water demand hazards;

3) During the past reference period (16:00 to 18:00)to have a water reserve during the DR event (18:00 to 20:00).

4.3. DR Bids Strategies and Financial Analysis

Problem 1 was solved for a range of spot prices.The reported results are optimal DR powers maximizing the profitability of the water system while meeting all constraints.The net benefit was defined as the difference between spot price and compensation.Simulation results as well as net benefit ranges for winter 2016 between 18:00 to 20:00 are presented in Table 1.

As shown in Table 1, the NEBEF mechanism was not financially viable during 4.5% of the time as spot prices were less than the compensation price (negative net benefit).This implicitly implies that the power system did not need any DR because the available generation was sufficient to meet the requested demand at minimum cost.

Fig.4.Optimal energy consumption over the system.

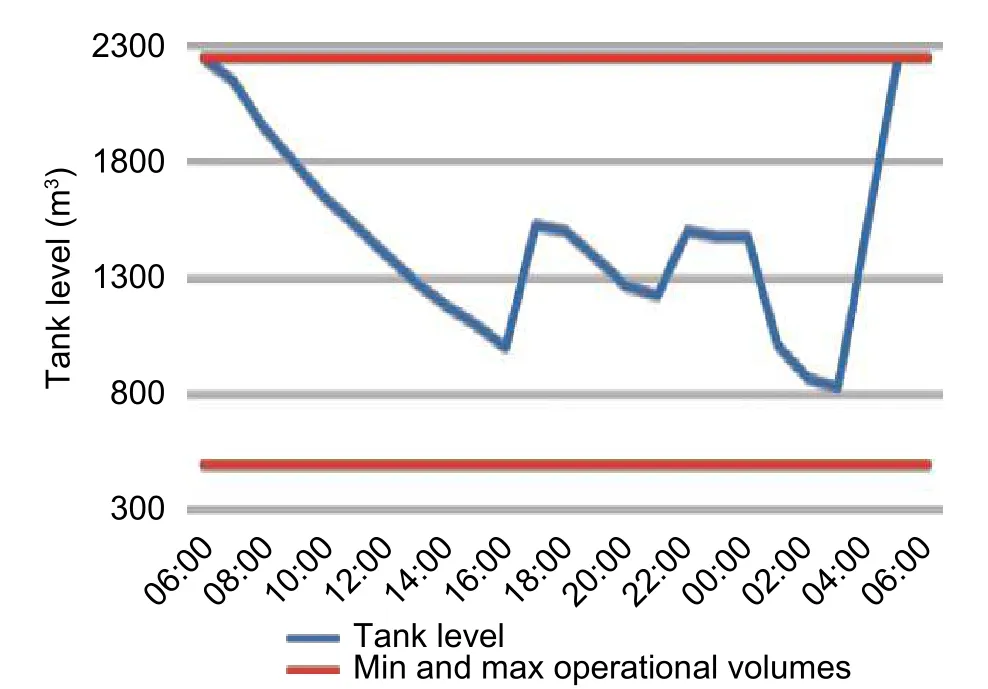
Fig.5.Tank level evolution with DR consideration.

For the positive net benefit ranges, the function of evolution of optimal DR power reduction is obviously growing with market price(see Fig.6).This is justified by the objective function, which aims to maximize the economic value of DR.The function is concave and the slope is decreasing with the price.The optimal DR power is:

1) Very sensitive for prices between 0 and 100 €/MWh since the water system still has an enough flexibility to react to the price signal;

2) Minimally sensitive for prices between 100 €/MWh to 400 €/MWh since the water system has only a reduced available flexibility;

3) Constant for prices ＞400 €/MWh as the water system is using its maximum DR power capacity.

On the other hand, the DR peak load reduction curve is compatible with the needs of the electric power system.The high price periods correspond to the most stressed supply/demand equilibrium periods on the market, when high-cost high-emissions fossil generation units are the most solicited and when DR is the most useful.

For financial analysis and for all market price scenarios considered for winter 2016, we compared:

1) The economic cost of a pump-scheduling day without DR consideration, which corresponds to the classical pump scheduling problem.

2) The economic cost of a pump-scheduling day with DR participation, where the economic cost corresponds to the pumping cost minus the DR economic benefits and depends on the market price scenarios considered.

The aim of this comparative study was to highlight the economic interest that DR through the NEBEF mechanism could bring to water utilities.For this purpose, two Monte-Carlo simulations were used to find the average strategic behavior of the water system regarding bids on the spot market.We considered market prices for winter 2016 on working days, corresponding to a total of 62 scenarios.Two Monte-Carlo simulations were performed:

1) Simulation 1: Removing five scenarios from November 2016 with price spikes above 250 €/MWh (57 scenarios).These scenarios were removed because they corresponded to the extreme and rare situations where the power grid was on the edge of stress.The results of this simulation would constitute a lower bound of the expected results.

2) Simulation 2: Considering all price scenarios from winter 2016 (62 scenarios).The results of this simulation would constitute an upper bound of the expected results.

The average economic gain and the average % gain are, respectively, defined as the difference and the relative difference between the optimal pump scheduling cost without DR and the optimal pump scheduling cost with DR, including DR benefits.Table 2 summarizes the numerical results obtained for the two simulations.

For the winter period studied, economic gains made by the water system would be in the interval [2.9%,3.2%] of its daily electricity bill.DR can thus be considered as a win-win alternative for both power system operators and water utilities.

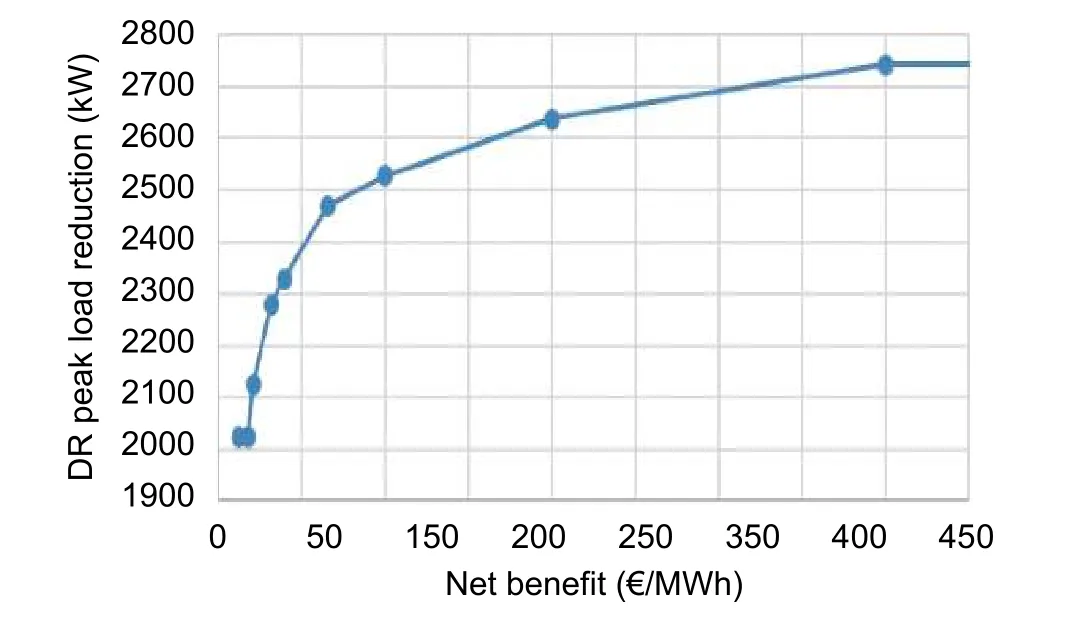
Fig.6.DR peak load reduction according to market price.

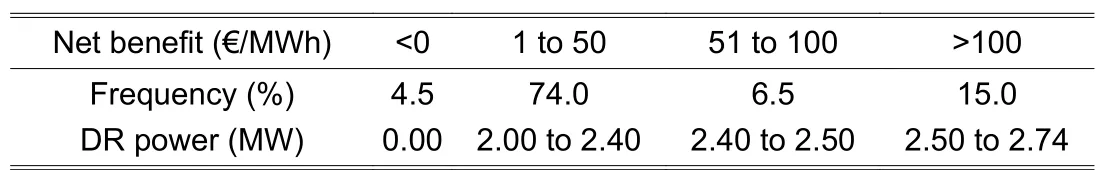
Table 1: Net benefit ranges frequency between18:00 and 20:00

Table 2: Monte-Carlo numerical results

4.4. Environmental Analysis

A major environmental challenge facing the world today is the risk of global warming.One of the major objectives of the energy transition is the reduction of greenhouse gas footprint, which is due in large part to the use of fossil power plants for electricity production.

France drives more than 72% of its electricity from nuclear energy, while fossil generation units account for only 9% of the country’s total electricity production[14], as shown in Fig.7.These fossil power plants, thanks to their great flexibilities that they can be activated very quickly, are considered as peak generation units and are used to respond to rapid changes in the electricity demands and to manage special consumption peaks.

The thermo-sensitive nature of the French electricity demand implies a strong solicitation of peak generation units in winter.Fig.8 shows the difference between CO2emissions per kWh produced on a winter and summer day, respectively[15].We observe that 42%more CO2is emitted in winter as compared to summer.

Taking into account compensation prices for 2016, a DR bid, if accepted in the market, would replace a peak generation unit bid according to the market’s merit-order principle (bids are accepted in an ascending order according to their operating costs).Renewables have a very low marginal cost and are found at the bottom of the market’s supply curve.Nuclear energy also has a low operating cost and follows the renewables in the ranking.Peak power plants, starting with coal-fired power plants,then combining with cycle gas plants (CCGT), and ending with diesel or gasoline fueled, have the highest running cost.Fig.9 illustrates the merit-order principle and shows how DR could replace peak generation production.

In the example of Fig.9, a peak day is considered with a compensation price of 56.1 €/MWh.Two supply curves on the market are considered: One with DR and one without DR consideration.In the situation without DR, block 4, corresponding to a combined cycle gas power generation bid, balanced the market with a marginal price of P＊.With DR consideration, the DR block 4’ put for sale with a price of P＊’＜P＊, replaced block 4 according to the merit-order principle and led to a new market price P＊’＜P＊.As shown in Fig.9, the DR block 4 would be inserted between two peak generation unit blocs, depending on the DR bid price and peak generations unit variable cost.In addition, it could also lower the market price if the DR bid is competitive (large volume).

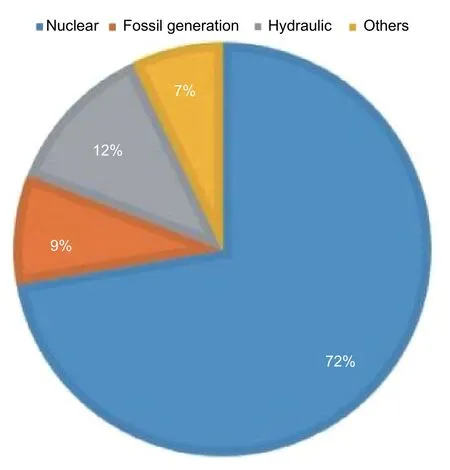
Fig.7.French power generation mix.

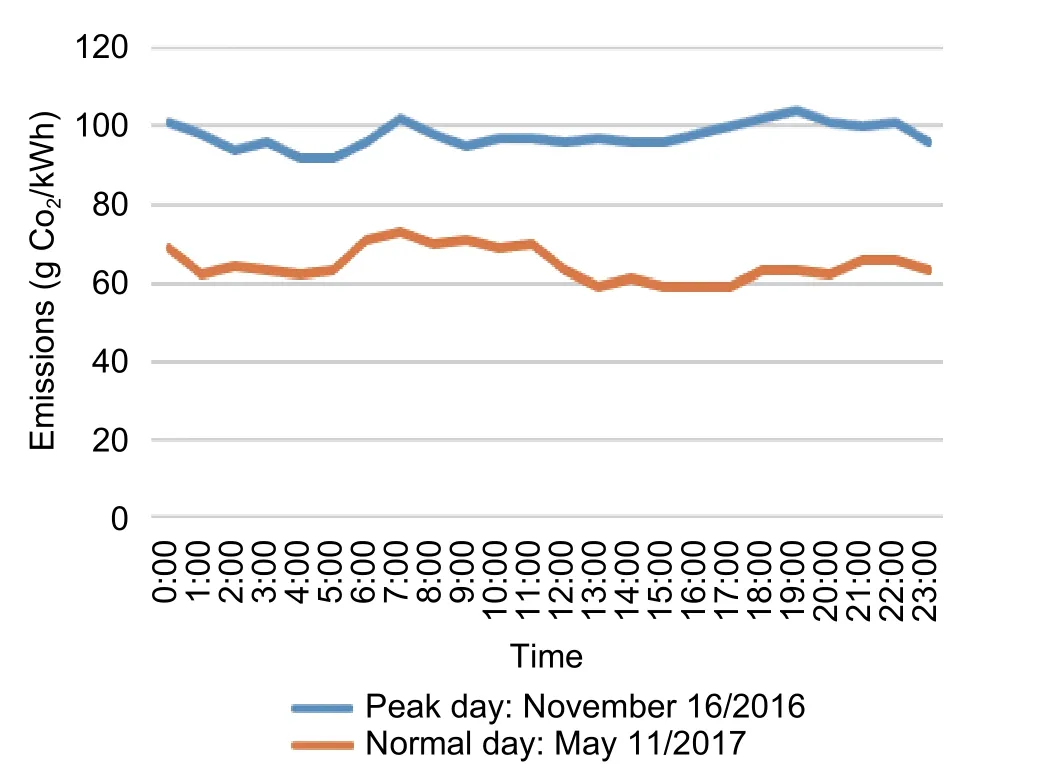
Fig.8.CO2 emissions for a normal and peak day (data source: RTE éCO2mix[15]).

For environmental analysis, Monte-Carlo simulation results from Table 2 were used to calculate the CO2savings for the system through peak load reduction.It is assumed that each DR bid sold on the market is a complete substitution to a peak generation production.Table 3 shows the average contributions of the French peak generation technologies to CO2emissions[15].Since it is difficult to estimate accurately the peak generation technology replaced by DR blocs, the average grams CO2/kWh (g CO2/kWH) ratio from all French peak generation technologies was used,weighted by their utilization rates during the year 2016[14].

Significant CO2reductions by the water system through peak load reduction during evening peak are highlighted in Table 4.The water system can reduce for up to 2.4 tons of CO2by day, which is the equivalent of the emission of 1600 cars during 10 kilometers of driving[16].

4.5. French Extrapolation

To give more significance to the previous results,we propose to estimate the DR NEBEF potential at the French scale.The approach considered consists in extrapolating the results obtained from the system studied to all the French water systems, assuming they all have a comparable flexibility.Indeed, a water system’s flexibility depends on the profile of its water demand, the characteristics of its equipment (storage tanks and pumps), and the nature of its topology.However, because tanks are sized to cover summer water demand peaks, they have an extra reserve margin in winter.This extra reserve margin can be optimized for DR operations, which justifies the assumption of a comparable flexibility of French water systems in winter.

In France, the average daily consumption of a person is around 140 liters[17], which gives, for the whole country,a daily consumption of around 9100000 m3.Previous results obtained on the system with a demand of 50000 m3were extrapolated to a water demand of 9100000 m3as shown in Table 5.

Results shown in Table 5 demonstrate that water utilities can trade DR on the French spot market while generating significant economic gains on their electricity bill.In addition, the aggregation of the water systems’ flexibility in France can reduce the power up to 450 MW during winter peaks.This peak power reduction, even if it is small in the French system where the peak load amounts to 90000 MW, can be very important in a period of stress on the power system.Indeed, the example of the crisis in California in June 2000 illustrates this fact: Rolling blackouts had occurred due to a shortage of 300 MW in a system of 50000 MW[18].Furthermore, many CO2emissions could also be saved by avoiding the production of additional electricity coming from fossil generation units to balance the supply and demand on the market.

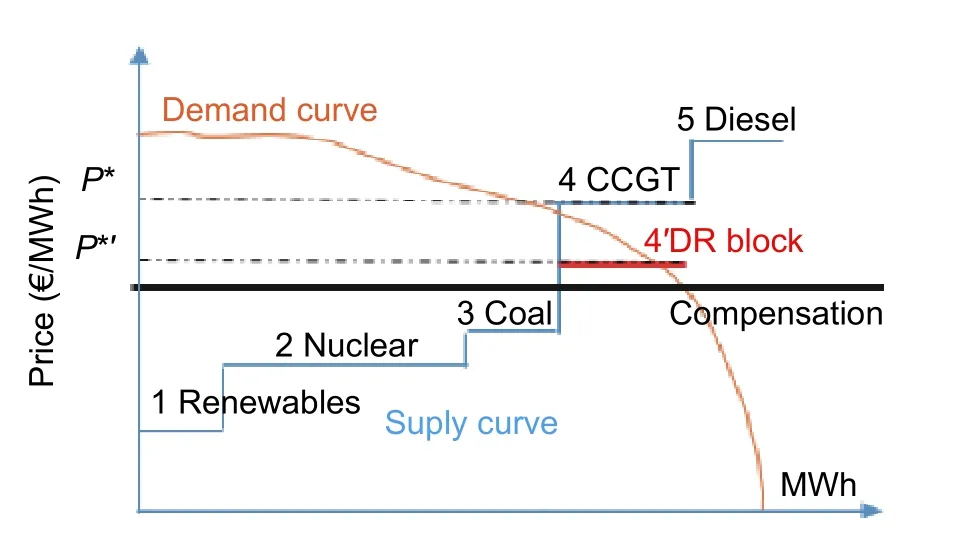
Fig.9.Merit order principle and DR impact on the supply curve.

Table 3: Contribution of French fossil power plants to CO2 emissions in grams CO2 (source RTE éco2mix)

Table 4: CO2 savings by the water system through DR participation

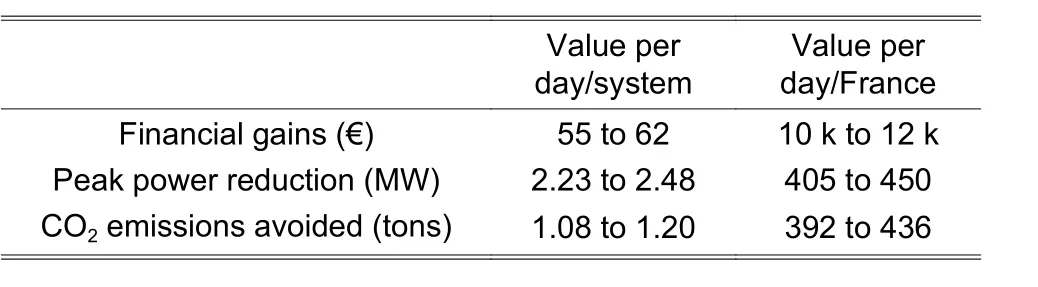
Table 5: NEBEF potential for water systems in France

4.6. Discussion and Future Work

The development of demand side management in the industrial sector could be hampered by two obstacles:Financial viability and risk management[19].Companies could be reluctant to participate in DR programs if they do not well manage the uncertainties and risks about the operation of their systems.Meanwhile, they must ensure a sufficient financial viability for the DR participation to remain competitive in markets.Mathematical programming could address both of these challenges, as shown in this article for the water industry where the case of a medium size water system was discussed.The mathematical model makes it possible to:

1) Estimate, based on a water demand history, the extreme water demands with a certain degree of robustness;

2) Secure the operation of the system regarding water demand hazards by keeping an extra water volume margin in tanks (optimization of tank level management);

3) Optimize DR load reduction powers by scheduling pumps.

Numerical results were discussed considering French spot prices for the winter 2016.Two Monte-Carlo simulations were performed, corresponding to low and high averages of the expected results.The results were discussed under three criteria:

1) Operational criterion: Operating the water system;

2) Economic criterion: % of gains for the water utility;

3) Ecological criterion: DR load reduction powers by scheduling pumps.

Benefits have been demonstrated considering these three criteria, confirming the relevance of the approach.However, the bold extrapolation made in subsection 4.5 may be questionable.Indeed, the hypothesis of a comparable flexibility for all French systems in winter is based on the fact of a better flexibility of tanks in winter due to a lower water demand.However, other parameters have not been taken into consideration such as the nature of pumps and the topography of the system.

Finally, it would be interesting to aggregate the flexibility of several water systems to propose large volumes of peak load reduction, enabling to improve the power system’s reliability at peak times.The difficulty would be to manage several independent systems through a mathematical optimization model.Information exchange between different water systems and the joint management of uncertainties are two challenges to be tackled.

5. Conclusions

The presence of storage units such as tanks and reservoirs gives drinking water systems a flexibility that can be integrated in power system operation through DR.The use of mathematical programming can enable water system operators to participate in DR mechanisms such as the NEBEF mechanism, making it possible to reduce peak energy load and CO2emissions while generating economic gains on water utilities electricity bills.Moreover, taking into account uncertainties about water demands in the mathematical model secures the operation of water systems in real time regarding hazards about water demands.Combination of these elements (mathematical programming and uncertainties) could give water utilities more confidence to participate in efficient DR programs.