基于加速度反演频域动载荷的一种正则化途径

彭凡+王樑+肖健+胡绚+韦冰峰2

摘 要：基于加速度频响函数矩阵反演频域动载荷是病态逆问题，反求的结果精度差，对数据的小扰动敏感，基于Tikhonov正则化方法，提出一种反演途径，将测点响应与待求激励进行归一化变换，在此基础上引入变换后的频响函数矩阵和正则化泛函进行求解，应用广义交叉验证准则选取最优正则化参数.考虑简支矩形薄板上的4个动载荷的识别问题，分析激励点和响应测点的不同位置以及动载荷大小之间相差程度不同的4个算例，将本文方法与不采用归一化变换的正则化求解结果进行2种相对误差的均方根比较.结果表明，利用归一化变换可提高动载荷反演精度，增强正则化方法的抗噪能力，当测点之间的响应以及各动载荷大小相差较大时，明显改善了识别精度.

关键词：动态载荷；频响函数；反问题；正则化；归一化

中图分类号：O326；O347.1 文献标志码：A

A Regularization Approach of Dynamic Load Identification

in Frequency Domain by Acceleration Responses

PENG Fan1 ，WANG Liang1，XIAO Jian2，HU Xuan1 ， WEI Bingfeng2

（ 1. College of Mechanical and Vehicle Engineering， Hunan University， Changsha 410082， China；

2. Research Institute of Beijing Structure and Environment Engineering，Beijing 100076， China）

Abstract：Load identification based on acceleration frequency response matrix is an ill-conditioned problem. The identification accuracy can obviously be affected by small perturbations of the response data. Based on Tikhonov regularization method， a new approach is proposed in which both the response data at measured points and the loads to be identified are normalized， the transformed frequency response matrix and regularization function are introduced， and the corresponding problem of functional minimum is solved to obtain the loads. The optimal regularization parameters are determined by generalized cross validation criterion. The identification of four transverse dynamic loads on a rectangular thin plate with simply supported edges is performed. Four numerical examples are designed to have different application locations of loads and measured points as well as different magnitude ratio of dynamic loads in frequency domain. The results show that the new approach of dynamic load identification in frequency domain is effective to improve the identification accuracy and the noise resistance. Particularly， the errors of the identification can be significantly reduced in the cases where the large difference between the magnitudes of dynamic loads in frequency domain exists， or when excitation positions are close to structural boundaries.

Key words：dynamic loads； frequency response function； inverse problem； regularization； normalization

載荷识别的理论和应用研究受到了研究者越来越多的重视[1-5]，目前，人们已提出了多种频域内反演动态载荷的技术，其中频响函数矩阵求逆是一类重要方法[6-7].然而，反演的病态特性使得量测数据的小扰动导致结果不准确，甚至不可信.当以测点的加速度频域响应作为输入时，低频段的反演误差非常明显.正则化方法是提高反求精度和稳健性的一条重要途径，典型的正则化方法包括截断奇异值分解和Tikhonov正则化方法[8].截断奇异值分解法的基本思想是将所得的广义解式子右端进行截断[9]，即只保留前面若干个对应于较大奇异值的部分，将后面的对应于较小奇异值的部分过滤掉，如何选取截断阈值，是该方法的难点.Tikhonov正则化方法通过引入包含响应残差和激励的模的泛函，由泛函对载荷的一阶偏导为零，得到正则化以后的激励求解列式[10-11].文献[12-13]提出综合使用奇异值分解法与Tikhonov正则化的载荷识别策略，当频响函数矩阵的条件数大于某一临界值时，使用正则化技术，反之，由奇异值分解法实施反求.张磊等[14]提出在总体最小二乘算法的基础上进行Tikhonov正则化，利用共轭梯度法解算该目标函数的最优化问题.然而，当各响应测点的响应之间、响应与激励之间以及激励与激励之间在数量上差别大，会导致正则化方法效果差，有必要重新考察变分泛函的构造.为此，文中由归一化变换使得加速度响应和激励的模值在一个相近的范围内变化，在此基础上重新构造变分泛函，给出一种Tikhonov正则化求解途径，通过简支矩形薄板的多点载荷反求算例检验其有效性.

2 算例及讨论

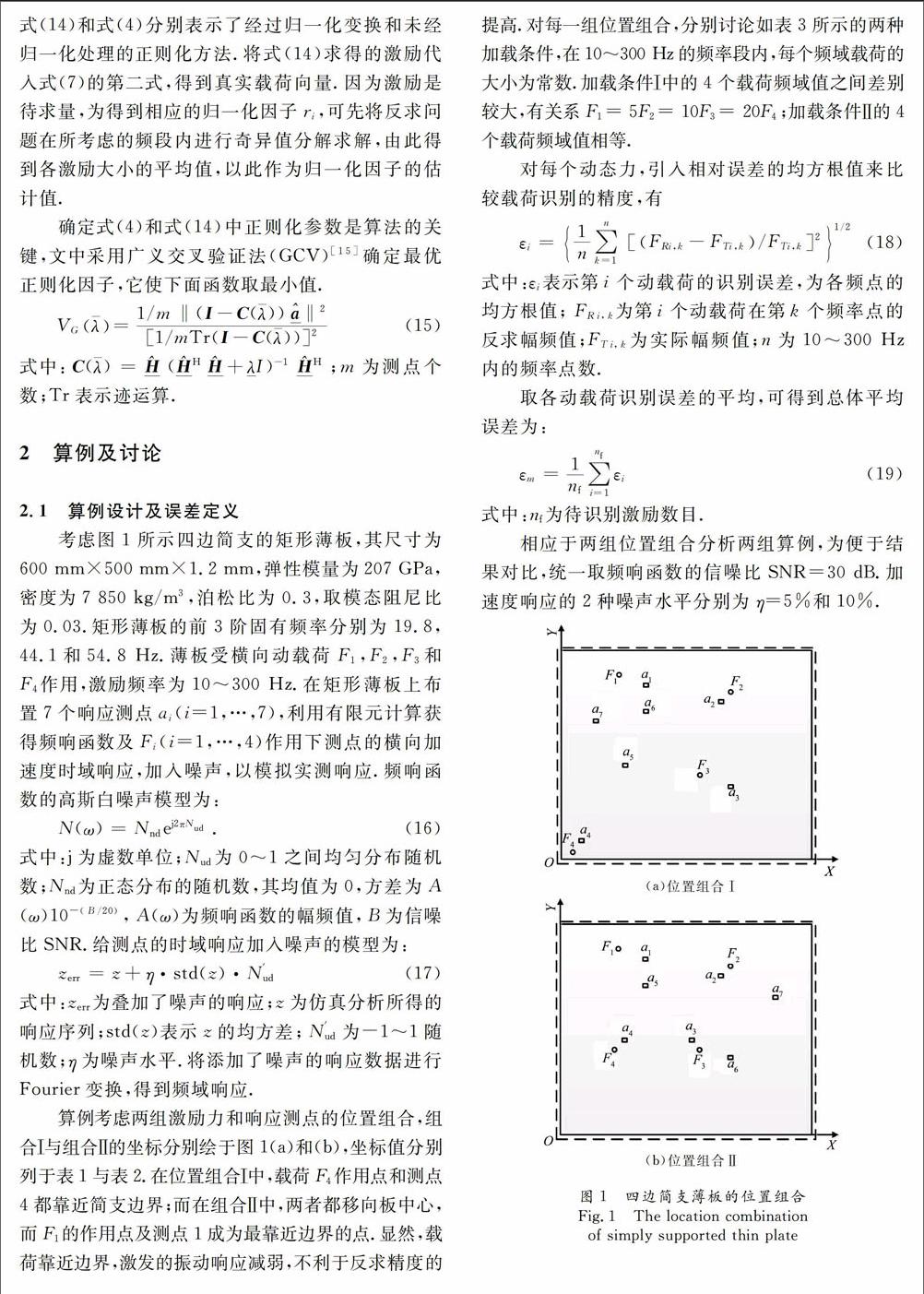
2.1 算例设计及误差定义

2.2 算例1

对于表1所示位置组合Ⅰ的动载荷，考虑加载条件Ⅰ，分别采用不经归一化处理和经过归一化处理的正则化方法反求载荷，结果如图2所示.由图2可知， 4个反演载荷均在低频范围内波动大，在较高频段内，反求值与实际值相差很小.这是因为基于加速度反求的逆运算项之模值随频率减小而显著增加，随频率增加而减小，故在低频段，频响函数矩阵求逆的条件数较大，导致较大的误差与波动.图2表明，归一化变换后的正则化求解能提高识别精度，尤其在低频段内，效果明显.而在大于二阶基频（约45 Hz）的频率段，两种反演途径给出的结果接近相同.

分析载荷频域值相同的加载条件Ⅱ，识别误差结果如表4所示.由表4可知，2种正则化途径所得结果的误差相比表3列出的对应值减小，归一化处理对F1，F2和F3的识别效果改善较小，但明显提高了F4的识别精度.这是因为F4接近边界，产生的测点加速度响应小，归一化变换将其影响放大了，使得其识别精度得到提高.

2.3 算例2

分析位置组合Ⅱ，首先考虑载荷条件Ⅰ，误差结果如表5所示.比较表5与表3可见， F4的误差减小，而F1的误差有所增加，主要原因是此位置组合中的F4作用点靠近板中部，从而激发了较强的响应，而F1相对其余3个载荷更靠近边界.归一化变换改善了反求的总体精度，且明显降低了F4的相对误差.

考虑载荷条件Ⅱ，误差如表6所示.从总体和个体来看，2种正则化途径所给结果的识别精度接近相同，原因在于4个频域载荷相同，且作用点离边界较远以及各测点频域相应的强度接近相同.同样由于F1离边界相对最近，故识别误差最大的载荷由F4变成了F1，而归一化处理使得F1的识别误差略有减小.

3 结 论

在频域中采用归一化变换，将各测点响应和待求载荷的大小变化调整到相近的范围，构造新的Tikhonov泛函进行正则化求解.利用数值仿真所得结果可知：

1）归一化处理能从整体和个体上提高各动载荷的反演精度.

2）当频域动载荷大小相差较明显，或者载荷作用点靠近边界时，归一化处理使得相应荷载的反求精度明显改善，抵抗测量噪性干扰的能力增强.

参考文献

[1] LNOUE H，HARRIGAN J J，REID S R. Review of inverse analysis for indirect measurement of impact force[J].Applied Mechanics Review，2001，54（6）：503-525.

[2] 韩旭，刘杰，李伟杰，等.时域内多源动态载荷的一种计算反求技术[J].力学学报，2009，41（4）：595-602.

HAN Xu， LIU Jie， LI Weijie，et al.A computational inverse technique for reconstruction of multisource loads in time domain[J].Chinese Journal of Theoretical and Applied Mechanics， 2009， 41（4）：595-602.（In Chinese）

[3] SU N， LIU J ，HAN X， et al. A new improved regularization method for dynamic load identification[J].Inverse Problem in Science and Engineering，2014，22（7）：1062-1076.

[4] 彭凡，馬庆镇，肖健，等.整体平动自由结构载荷时域识别技术研究[J]. 振动与冲击，2016，35（6）：95-99.

PENG Fan， MA Qingzhen， XIAO Jian， et al. Research on load identification in time domain for free structures with oval translation[J].Journal of Vibration and Shock，2016，35（6）：95-99.（In Chinese）

[5] 彭凡，王樑，肖健.质量时变系统的动载荷识别[J].湖南大学学报：自然科学版，2016，43（8）：52-56.

PENG Fan， WANG Liang， XIAO Jian. Identification of dynamic loads in mass-variable system[J]. Journal of Hunan University：Natural Sciences， 2016， 43（8）：52-56.（In Chinese）

[6] SANCHEZ J， BENAROYA H.Review of force reconstruction techniques[J]. Journal of Sound and Vibration，2014， 333（14）： 2999 -3018.

[7] 刘恒春，朱德懋，孙久厚.振动载荷识别的奇异值分解法[J].振动工程学报，1990，3（1）：24-33.

LIU Hengchun， ZHU Demao，SUN Jiuhou.A singular value decomposition method for the identification vibration loads[J]. Journal of Vibration Engineering，1990，3（1）：24-33.（In Chinese）

[8] NELSON P A，YOON S H. Estimation of acoustic source strength by inverse of methods： partⅠ，conditioning of the inverse problem[J]. Journal of Sound and Vibration， 2000， 233（4）：643-668.

[9] THITE A N，THOMPSON D J.The quantification of structure structure borne transmission paths by inverse methods. part1： improved singular value rejection methods[J]. Journal of Sound and Vibration， 2003，264（2/3）： 411-431.

[10]KIM Y， NELSON P A. Optimal regularisation for acoustic source reconstruction by inverse methods[J]. Journal of Sound and Vibration，2004， 275（3/5）：463-487.

[11]CHOI H G，THITE A N，THOMPSON D J. Comparison of methods for parameter selection in Tikhonov regularization with application to inverse force determination [J]. Journal of Sound and Vibration， 2007，304（3/5）：894-917.

[12]CHOI H G， THITE A N， THOMPSON D J. A threshold for the use of Tikhonov regularization in inverse force determination[J]. Applied Acoustics， 2006，67（8）：700-719.

[13]郭榮，房怀庆，裘剡，等.基于Tikhonov正则化及奇异值分解的载荷识别方法[J].振动与冲击，2014，33（6）：53-58.

GUO Rong， FANG Huaiqing，QIU Shan，et al. Novel load identification method based on the combination of Tikhonov regularization and singular value decomposition[J].Journal of Vibration and Shock，2014， 33（6）：53-58 .（In Chinese）

[14]张磊，曹跃云，杨自春，等.总体最小二乘正则化算法的载荷识别[J]. 振动与冲击， 2014，33（9）：159-164.

ZHANG Lei， CAO Yueyun， YANG Zichun，et al.Load identification using CG-TLS regulariztion algorithm[J]. Journal of Vibration and Shock，2014，33（9）：159-164.（In Chinese）

[15]GOLUB G H， HEATH M， WAHBA G. Generalized Cross-Validation as a method for choosing a good ridge parameter [J]. Technometrics， 1979，21（2）： 215-223.