一类相同材料周期焊接问题的稳定性

罗 文

(福建商学院 基础部，福建 福州 350012)



一类相同材料周期焊接问题的稳定性

罗 文

(福建商学院 基础部，福建 福州 350012)

文章讨论了同一材料在平面弹性中的周期焊接问题，通过引入两个全纯函数，将问题转化为周期黎曼边值问题。通过应力函数的表达式，并使用柯西型积分，研究了周期焊接问题的稳定性。

应力函数；周期焊接问题；摄动；稳定性

平面弹性材料的焊接问题在工程上具有广泛的应用，以复变函数为理论工具研究平面弹性问题是个很好的方法。

1.引言

假设Γ∈C是一条简单光滑的封闭曲线，以反时针方向为正向，Γ所围成的内部区域(外部区域)，记为D+(D-)。建立函数关系：

Γδ∶ξ=α(t)=t+δ(t),t∈Γ

(1.1)

当δ∈C′(Γ)时，容易得到：

(1.2)

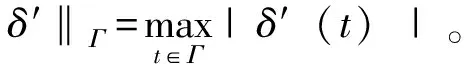
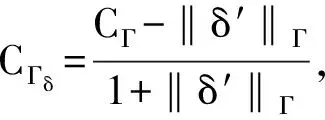
对于平面弹性基本问题[1-2]，弹性体内任意点z处的应力σx,σy,τxy和位移u+iv，可以由应力函数φ(z),ψ(z)表示为：

(1.3)

其中μ,κ为弹性常数。

2.周期焊接问题

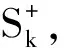
问题Ⅰ：设在一个弹性平面中有一列周期孔L0,L±1,L±2，…，以aπ为周期，在各孔上焊接材料相同的垫圈，设孔边和垫圈线间的位置差异已知，则：

(u++iv+)-(u-+iv-)=g(t),t∈L

其中g(t)也以aπ为周期，并且g′(t)∈H，在z=±∞i处的外应力为零。

3.摄动后的周期焊接问题

那么，称f*为f关于δ的指数为ε的n阶Sobolev型摄动，简记为Φ*∈sp(Φ,δ,ε,n)

假设焊接中问题Ⅰ的孔边和垫圈间的位置差g(t)产生误差，该误差可以看成定义3.1中的Sobolev型摄动，此时的焊接问题就是摄动后的焊接问题，即问题Ⅱ：

在一个弹性平面中有一列周期孔L0δ,L±1δ,L±2δ，…，以aπ为周期，在各孔上焊接材料相同的垫圈，该孔边和垫圈线间的位置异为：

(3.1)

其中gδ(ξ)也以aπ为周期，并且gi(ξ)∈H,i=0,1,2，在z=±∞i处的外应力为零。

根据焊接条件，问题Ⅱ的应力函数

(3.2)

(3.3)

联立(3.2)与(3.3)可以得到：

(3.4)

(3.5)

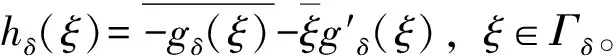
(3.6)

(3.7)

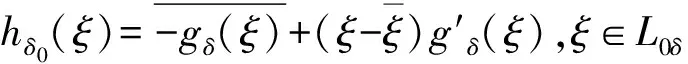
(3.6)和(3.7)是周期跳跃问题，其解分别为：

(3.8)

(3.9)

由于所讨论的焊接问题要求在Z=±∞i的外应力为零，所以可以得到焊接问题Ⅱ的应力函数为：

φδ(z)=φδ0(z),Z∈S0δ/L0δ

(3.10)

(3.11)

4.周期焊接问题的稳定性

4.1 几个引理

为了讨论应力函数的稳定性，需要以下几个引理：

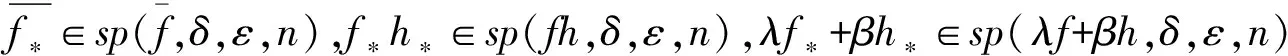
引理2：记柯西积分算子为：

与之相应的射形算子为：

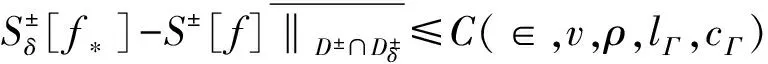
4.2 周期焊接应力的稳定性

根据(1.1)中Γδ的定义可知，如果δ≡0,t∈Γ，则Γδ=Γ，即不发生摄动，若焊接问题Ⅱ中δ=0，则可转化为焊接问题Ⅰ，因此令δ≡0，从(3.10)，(3.11)可得周期焊接问题Ⅰ的应力函数为

φ(z)=φ0(z),z∈S/L

(4.1)

(4.2)

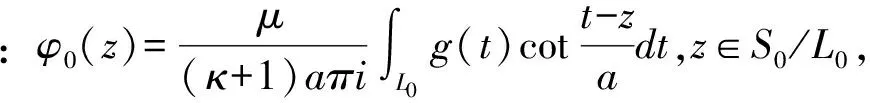
定理1 假设Γδ∈P(Γ,‖δ‖<ρ)，g(i)∈sp(g(i),δ,∈,0),i=0,1那么:

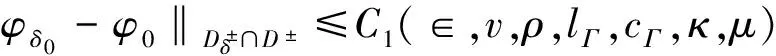
(4.3)

(4.4)

其中Ci(∈,v,ρ,lΓ,cΓ,κ,μ),i=1,2是不依赖于δ的常数。

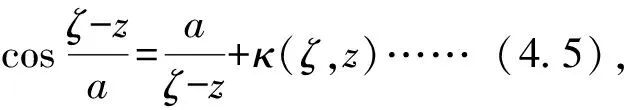
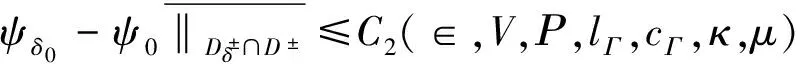
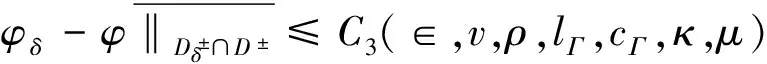
(4.6)

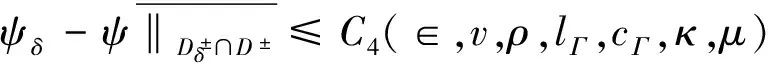
(4.7)

其中Ci(∈,v,ρ,cΓ,κ,μ),i=3,4是不依赖于δ的常数。

[1]Lu J K.Complex variable methods in plane elasticity[M].ingapore: World Scientific，1995.

[2]Muskhelishvili N I.Some basic problems of the mathematical theory of elasticity[M].Leyden：P.Noordhoff，1963.

[3]Lin Juan，Duan Ping，Xie Bihua.Stability of a kind of welding problems of infinite plane with a unit circular[J].Journal of Mathematics，2013，33(6):969-976.

[4]Juan Lin，Jinyuan Du.Stability of displacement to second fundamental problem in plane elasticity[J].Acta Mathematica Scientia，2014，34B(1):125-140.

[5]Pei Dang，Juan Lin，Jin-Yuan Du.Stability on a kind of welding problems under perturbations[J]. Applicable Analysis，2014，93(11):2478-2497.

(责任编辑：杨成平)

Stability of Periodic Welding Problem for the Same Material

LUO Wen

(Department of Foundation , Fujian Commercial College, Fuzhou 350012, China)

This paper discusses periodic welding problem for the same material in plane elasticity. By introducing two holomorphic functions, periodic Riemann boundary value is studied; by introducing stress function and Cauchy’s integral formula, the stability of the periodic welding problem is studied.

stress function; periodic welding problem; perturbation; stability

2016-11-04

罗文(1982—)， 男，福建尤溪人，讲师，硕士。研究方向：基础数学。

O17

A

1008-4940(2016)06-0105-04