一类具有强阻尼的高阶Kirchhoff型方程的初边值问题

晋守博

（宿州学院数学与统计学院，安徽 宿州 234000）

一类具有强阻尼的高阶Kirchhoff型方程的初边值问题

晋守博

（宿州学院数学与统计学院，安徽 宿州 234000）

针对一类具有强阻尼项的高阶Kirchhoff型方程的初边值问题，首先引入了几个重要的泛函和集合，并给出了整体弱解的定义。然后通过两个引理分析了整体弱解的性质，并且使用Galerkin方法构造了高阶Kirchhoff型方程的近似解。最后利用一些先验估计式，分析了近似解在不同范数下的有界性和收敛性，证明了这些近似解的极限就是高阶Kirchhoff型方程的整体弱解。

高阶Kirchhoff型方程；强阻尼；整体弱解；Galerkin方法

1 引言

本文将考虑如下一类具有强阻尼的高阶KirchhoffΔ型方程的初边值问题

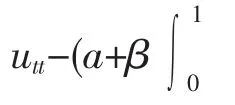
2 主要引理

首先给出所需的数学符号如下：

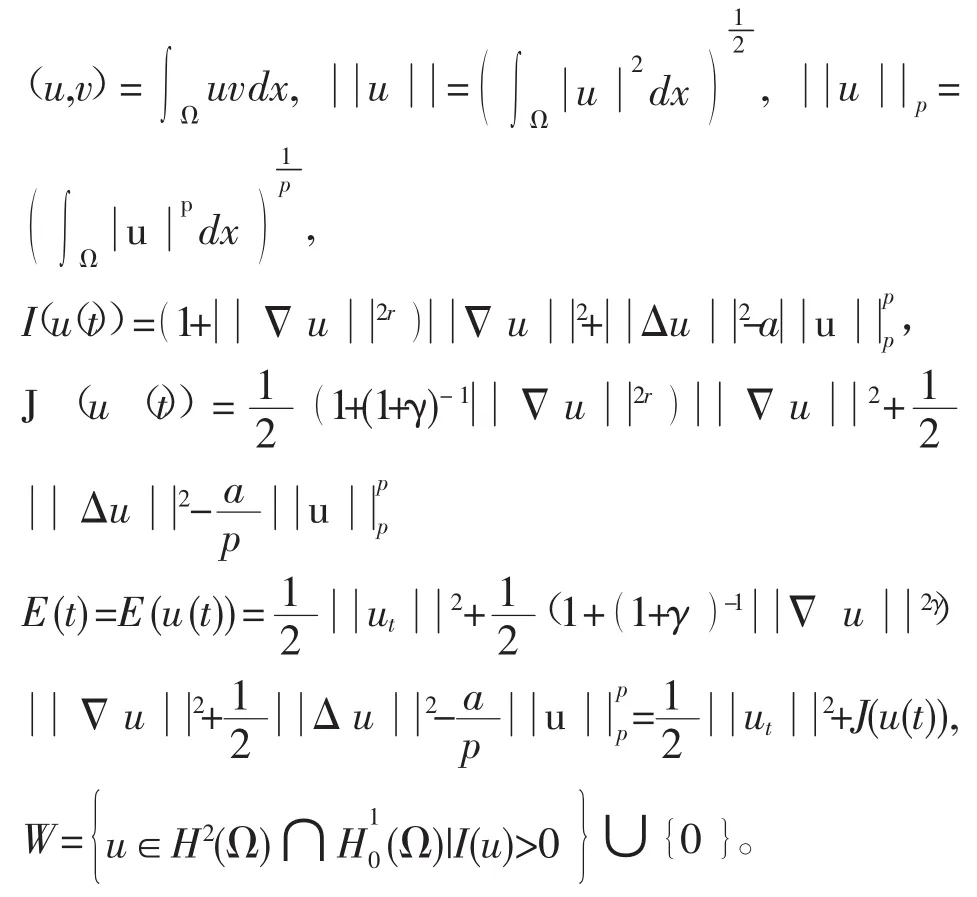
在区间[0,T）上给出初边值问题(1)整体弱解的定义，其中为任意正常数或者正无穷大。

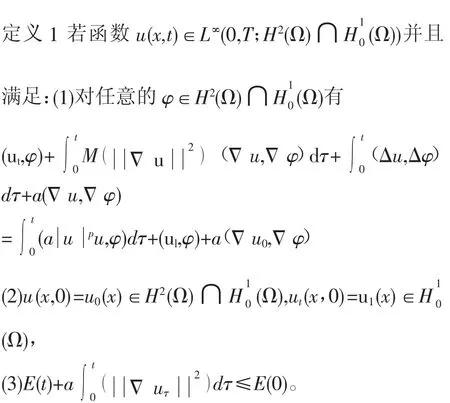
现在给出本文所要用到的主要引理。

引理1若u(x,t)为方程(1)的整体弱解，则能量泛函E(t)满足：

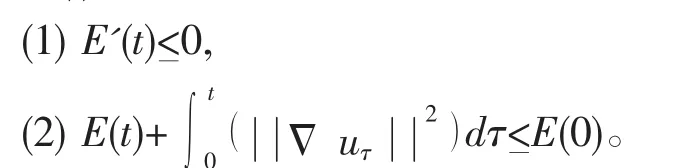
证明：仿照文献①中引理2.1的证法可以得出结论。

3 整体弱解的存在性

在这一部分，将给出方程(1)整体弱解的存在性。

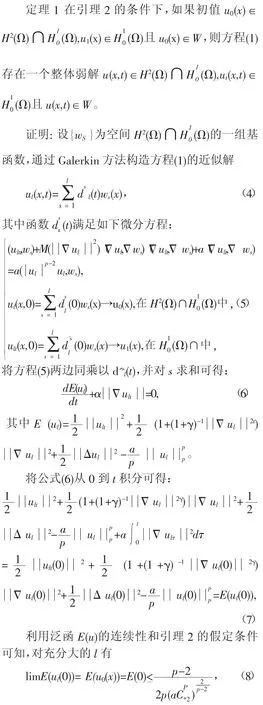
根据文献①Lions J L.Quelques méthodes de résolution des problémes aux limites non linéaires,Dounod Gauthier Villars,Paris,1969.第一章引理1.3，可以得到

The initial boundary value of the higher order Kirchhoff type equation with strong damping

JIN Shoubo

In this paper,the initial boundary value of the high order Kirchhoff type equation was studied.Firstly,we introduced some important functional and sets,defined the global weak solution,and analyzed the property of the global weak solutions through two lemmas.Then the approximate solutions of the higher order Kirchhoff type equation were constructed.Finally,the bounded property of the approximate solutions was analyzed by some priori estimates in different norm,and the convergence of the approximate solutions was discussed.It is proved that the limit of the approximate solutions is a global weak solution of the higher order Kirchhoff type equation.

higher order Kirchhoff type equation；strong damping；global weak solution；Galerkin method

O175.4

A

1009-9530（2016）05-0096-04

2016-04-26

安徽省自然科学研究面上项目“分数阶微分发展系统的可控性研究”(1508085MA10)；宿州学院优秀青年人才支持计划重点项目“阻尼项和多个非线性源项共同作用下的波动方程整体解的存在性与不存在性问题”(2016XQNRL003)

晋守博(1980-),男，宿州学院数学与统计学院讲师,理学硕士,主要研究方向为偏微分方程。