On Collision Local Time of Two Independent Subfractional Brownian Motions

Jingjun Guo and Yanping Xiao

1 Introduction

Stochastic processes can make models in Biology,Physics,Engineering and so on,which have become an important tool to master for scientific and technological workers.In this paper,we mainly consider a self-similar Gaussian processsubfractional Brownian motion(sfBm).Let(i=1,2)be two independent subfractional Brownian motions(sfBms)with different parameterkion

For eachi=1,2,isa centered Gaussian process with representation

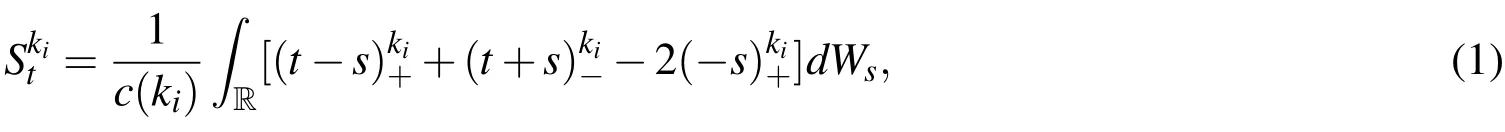
andWis a Brownian motion(Bm),which means that sf Bm is an extension of Bm,a rather special class of self-similar Gaussian process.

The object of study in this paper will be collision local time ofSk1andSk2,whichis formally defined as

whereδ(x)isa Dirac delta function,I=[0,T]andT＞0.The intuitive idea of the local timeLkfor two processes(i=1,2)is thatLkcharacterizes collision time during the interval[0,T].

Forki=0(i=1,2),processesSk1andSk2are classical Brownian motions(Bms).The local time of Bm has been studied by many authors.In recent years,some authors focus on the research on fractional integral process and related problems,e.g.fractional Brownian motion(fBm),due to its interesting properties and its applications in various scientific areas such as telecommunications,turbulence and finance[Biagin et.al(2008)].Others have studied the numerical solution of fractional-integral differential equations[Chen et.al(2014);Wang et.al(2015)].In general,sf Bm is intermediate between Bm and f Bm.The local times of sf Bm have been studied by many authors as well,e.g.[Liu et.al(2012)]for the intersection case and[Yan et.al(2010)]for the collision case,where author shave proved that the local time is smooth in the sense of Meyer and Watanabe.

In spite of sfBm has many properties analogous of fBm such as self-similar andlong-rang dependent, sfBm has non-stationary increments and weakly correlatedin comparing with fBm. On account of the complexity structure of sfBm, peoplepay little attention on these process. Owing to sfBm be not semimartingale(or Markov process), many classical methods in stochastic analysis can not dealwith the problems of sfBm. If we can get a continuous version of sfBm, it willbe effective to study the existence local time of sfBm through white noise analysisapproach. White noise is an original acoustic concept. In engineering technology,engineers often use the term of white noise to represent a kind of random disturbancein the dynamic system. For a long time, in order to give strict and reasonablemathematical meaning of white noise, Hida put forward infinite dimensional distributiontheory similar to Schwart distribution theory -white noise analysis. Nowwhite noise analysis becomes an effective method to deal with the problems ofinfinite dimensional space.

In this paper,motivated by[Oliveira et.al(2011);Liu et.al(2012)],we give an alternative expression of sfBm by using odd extension and fractional integrals operators,and study the existence of the collision local time of two independent sfBms with the different coefficients inWe prove that the collision time is a Hida distribution and belongs to(L2),respectively.The paper is organized as follows.In Section 2,we provide some background material from white noise analysis.In Section 3,we present the main results and their demonstrations.

2 White noise analysis

In this section, we briefly recall some notions and facts in white noise analysis, fordetails see Refs [Bender (2003); Biagin et. al (2008); Oliveira et. al (2011)].

The first real Gelfand triple is,whereandare the Schwartz spaces of the vector-valued test functions and tempered distributions,respectively.Denote the norm|·|inand the dual pairing betweenandby〈·,·〉,respectively.We consider two-independent d-tuple of Gaussian white noisesw=(w1,w2),wherewi=(wi,1,···,wi,d).For every test functionf=(f1,f2)on,the characteristic function of vector-valued whit enoisewisgiven by

Introduce the following notations:

n=(n1,···,nd),

LetΓ(A)be the second quantization ofA,whereAis defined byForeach integerp,letbe the completion of DomΓ(A)pwith respect to the Hilbert norm.Letbe the projective lim it ofand bethe inductive limit of,respectively.Thus,there is the second Gelfand triple:.Elements of(resp.∗)are called Hida testing(resp.generalized)functionals.For,theS-transform is defined by

Definition2.1.AmappingG:iscalledaU-functionalif

(1)G(λf1+f2)is entireinλforanypairof test functions;

(2)|G(zf)|≤C1expwithCi,p＞0,foranycomplexz.

Lemma2.2.LetdenoteasequenceofU-functionalwithfollowingprop-erties:

(1)forall,isaCauchysequence;

(2)thereexistCiandpsuchthat|Gk(zf)|≤C1expuniformlyin

Then,thereisauniquesuchthatS-1Gkconverges stronglytoΦ.

Lemma2.3.Letbeameasurespace,andletΦλbeamappingdefinedonΩwithvaluesin.WeassumeS-transformofΦ

(1)isanµ-measurablefunctionofλfor;and

(2)obeysaU-functional estimate

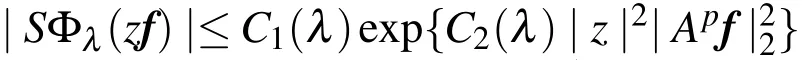
forsomefixedpandforC1∈L1(µ),C2∈L∞(µ).

Then

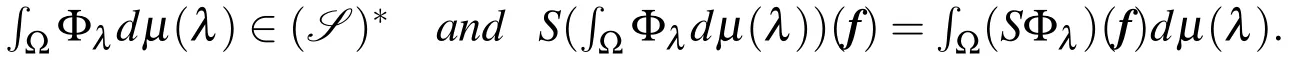
3 Collision local time

In this section,our main aim is to study the existence of the collision local time of two independentsfBmsSki={Ski(t),t≥0}(i=1,2)as(2).From now,we always approximate the Dirac delta function by the heatkernel

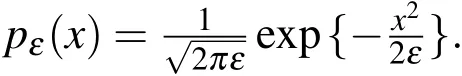
For anyε＞0,we define

To get ourmain results,we give an alternative expression of sfBmusing fractional integral soperatorsand odd extension.

Lemma3.1.Letbegiven.SubfractionalBrownian motionhasacontinuousversionofwheredenotestheoddextensionofand

proof:Forf:,we define its odd extensionfo(x)in[Tudor(2003)]as follows:

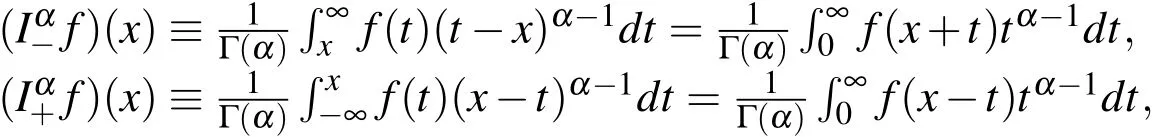
For givenα∈(0,1),we obtain if the integrals exist for allx.For an arbitrary parameter,using fractional integrals of Weyl’s typean alternative representation ofis given by

whereWis a Wiener process.Integrand in(5)yields

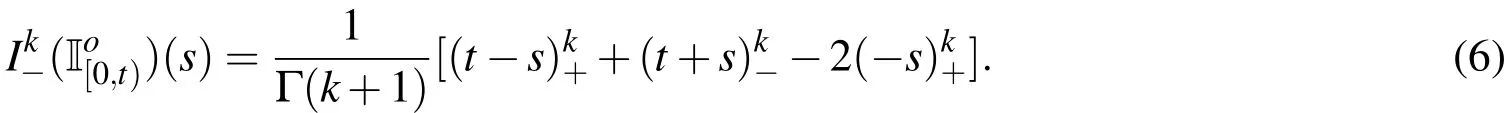
On the other hand,apart from fractional derivatives,we shall also use fractional derivatives operators.Forα∈(0,1)andε＞0,we have

The fractional derivatives of Marchaud’s type are given byif the integrals exist almost surely.In this case,the continuous version ofisUsingwe obtain the same form of continuous version.

From what we said above,we can safely see that as fBm is given by a continuous version offor

The following lemma is very useful to prove ourmain results.[Bender(2003);Drumond et al.(2008)]havegiven the similar estimate in discussion the local time of fBm,respective

Lemma 3.2.Letandbe given.Then there exists a non-negative constantCk such that

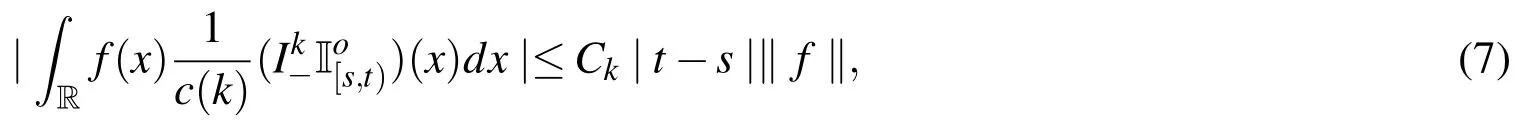
whereCk is some constant independent offand

proof:Recall thathas theaverage representation

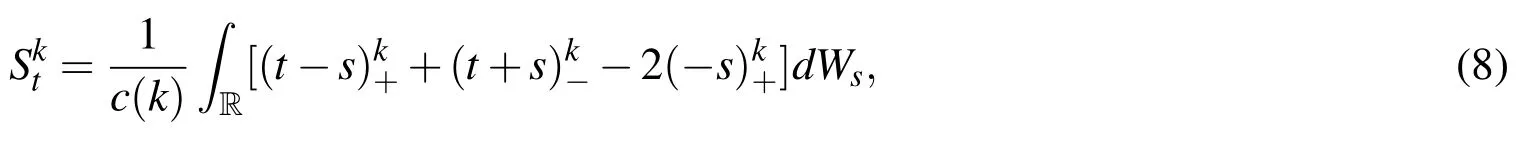
where{Wt}is a Bm andBy Lemma 3.1,(8)becomes

Fors≤tand givenwe obtain

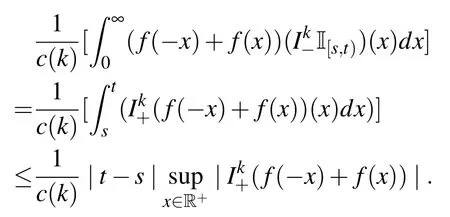
Next we will discuss the estimation of the integral for differentk.

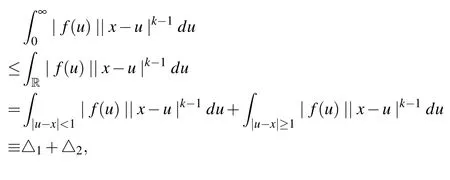
where

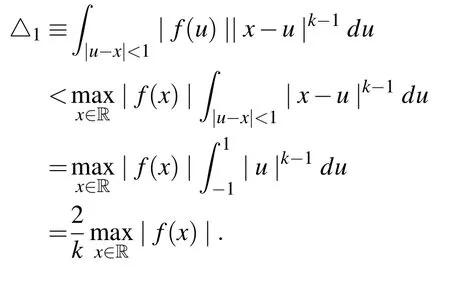
For the second part,wehave

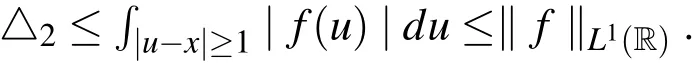
As similar techniques in the proof of Lemma 6 in[Drumond et.al(2008)]and applying Schwartz inequality,another integral becomes

Therefore

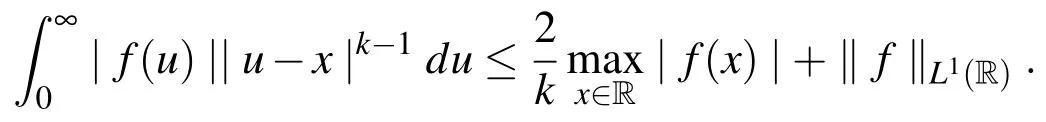
Nextusing similar methods,we estimate

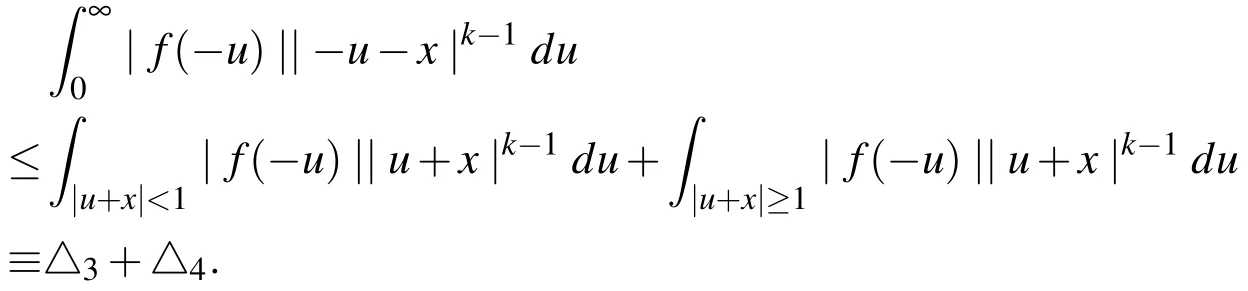
At the same time,we obtain

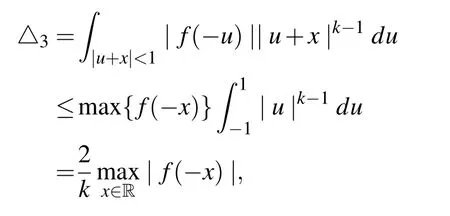
and

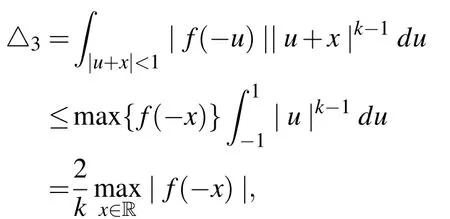
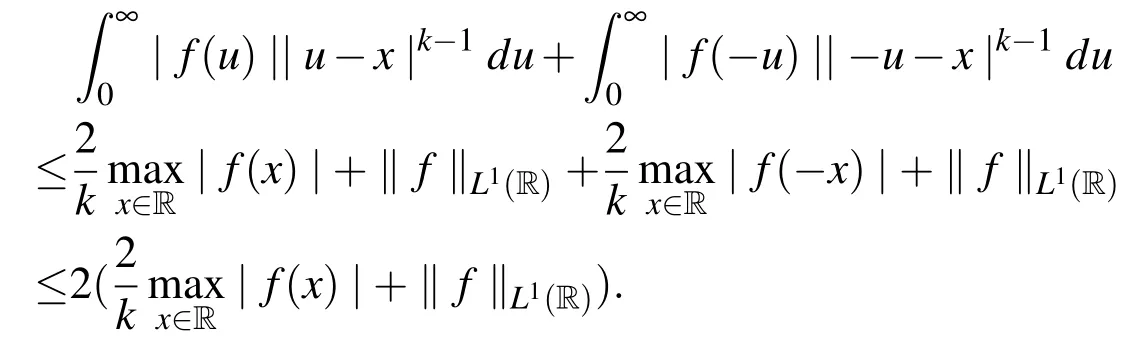
Hence

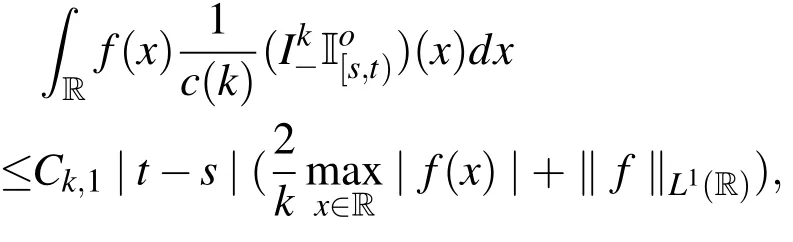
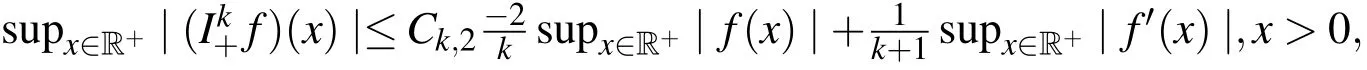
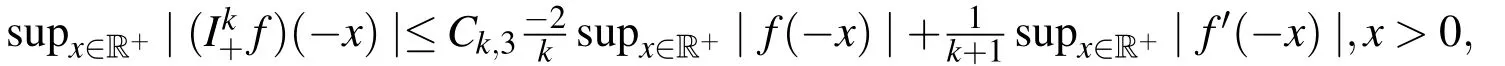
whereCk,2andCk,3are both constants de pendent on k.Thus we have shown that there exists a constantCk,4such that

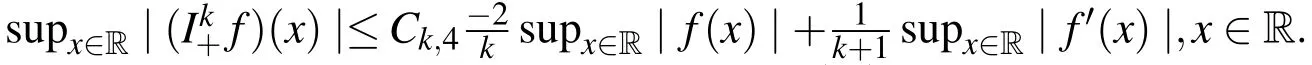
Therefore,for an arbitrary parameterwe obtain

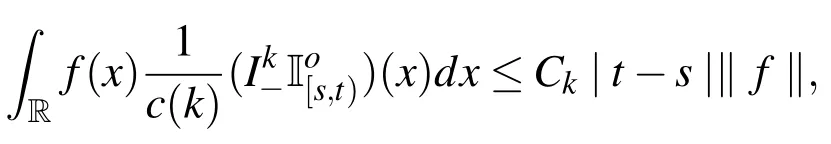
Theorem3.3.Foreachk1,k2andeverypositiveintegersuchthatd＜1,thecollisionlocaltimeoftwoindependentsfBmsSk1andSk2givenby

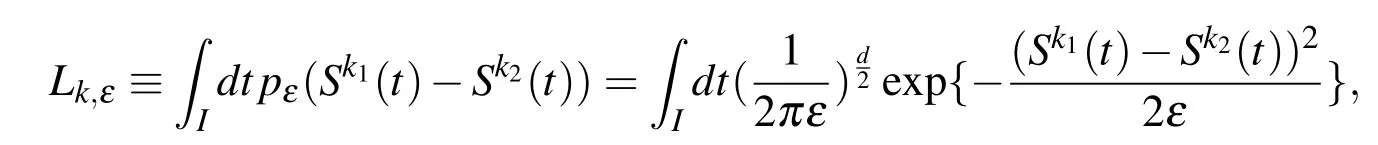
isaHidadistribution.Moreover,Lk,εhasthefollowingchaosexpansion

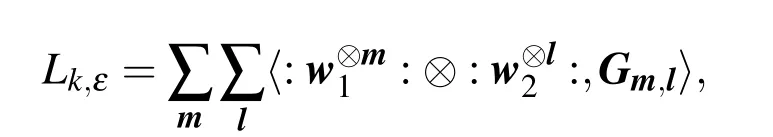
wherethekernelfunctions

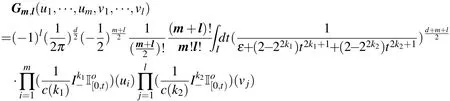
foreven,andzerootherwise.

proof:In order to prove the result,we only apply Lemma2.3 to theS-transform of the integral with respect to Lebesgue measuredtonI.

Denote

By the definition ofS-transform,we know that

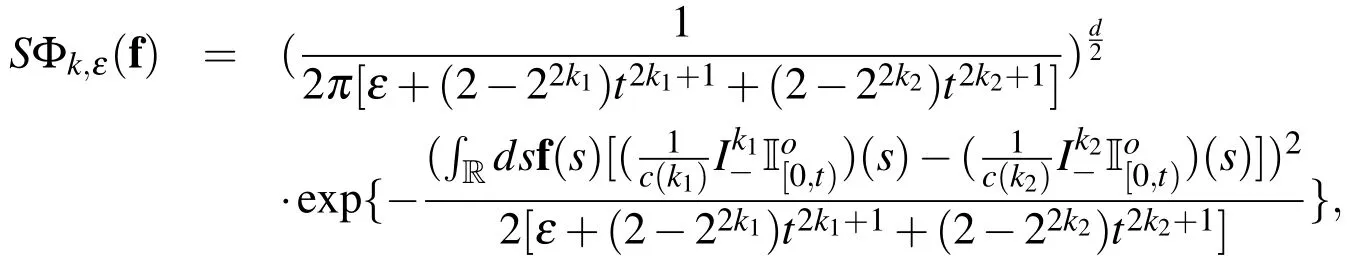
For any complex numberzand,it implies

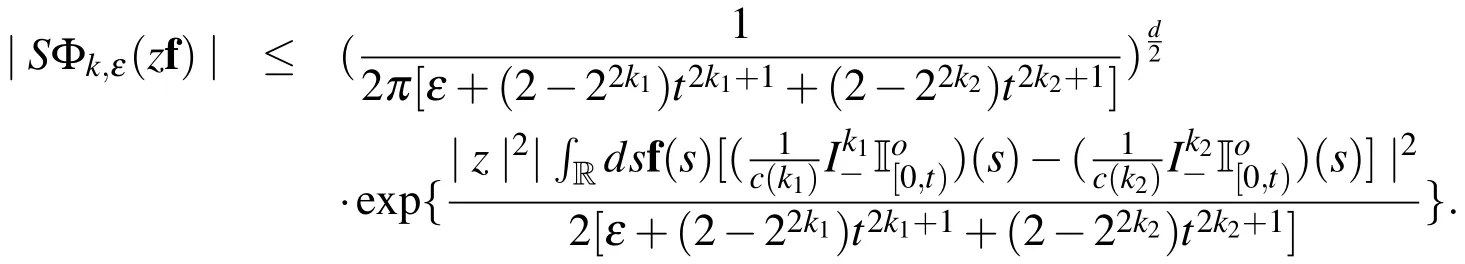
To check the boundary condition in Lemma 2.3,introduce norm‖·‖indefined by

By Lemma3.2we obtain

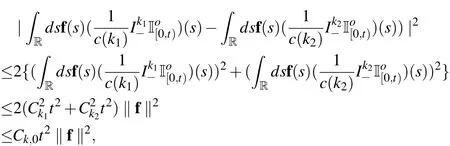
whereCk,0,Ck1andCk2arenotnegative constants.So

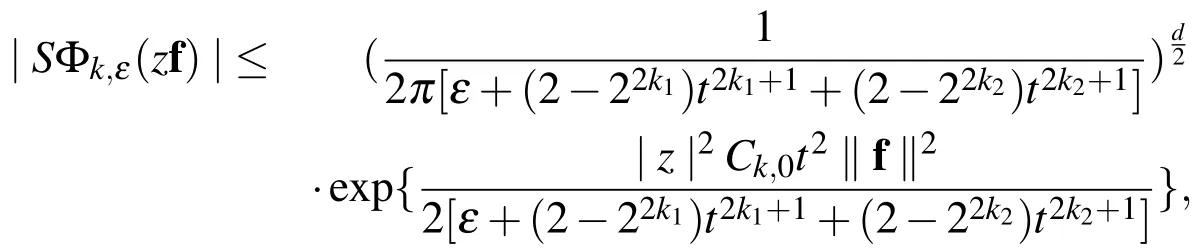
where the first part is integrableonIif(min,and thesecond part is bound.

To get the kernel functions ofGm,l,we consider theS-transform ofLH,ε.Comparing with the general form of the chaosexpansion,we find the kernel functions.

On account of the local time,it is desirable to be regularized by sequences of Gaussian,and renormalized functions of local time need be subtracted since the functions in hight dimensions fail to exist without subtractions[Nualartet.al(2007)].

We are now ready to prove that collision local timeLkis aswell as its subtracted counterpart,whereN≥0.

Proposition 3.4.Foreach k1,,every positive integerd≥1and N≥0such that(d+2N)min,the Bochner integer

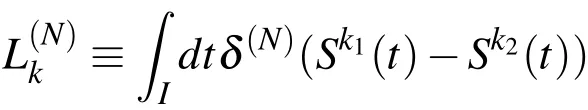
is a Hida distribution.

proof:Let us denote the truncated exponential series by expN(x)≡.For eacht＞0,the Bochner integral

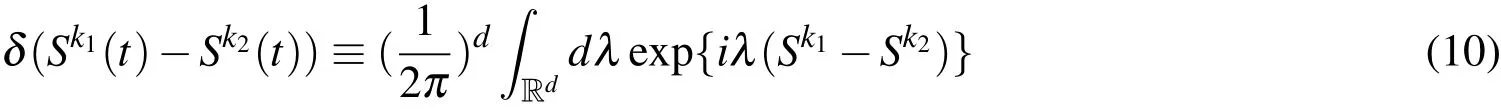
is a Hida distribution.ItsS-transform is given by

In fact,sinceSk1(t)andSk2(t)are independent of sfBms,then

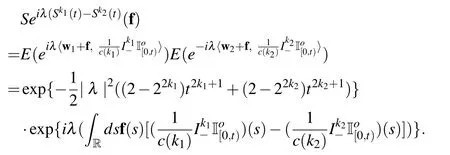
We can verity that theS-transform of the integrand satisfies the conditionsof Lemma 2.3.

Hence theS-transform ofδ(N)isgiven by

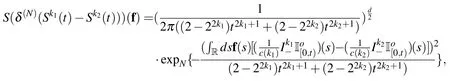
which is a measurable function.To prove the bounded condition,we consider

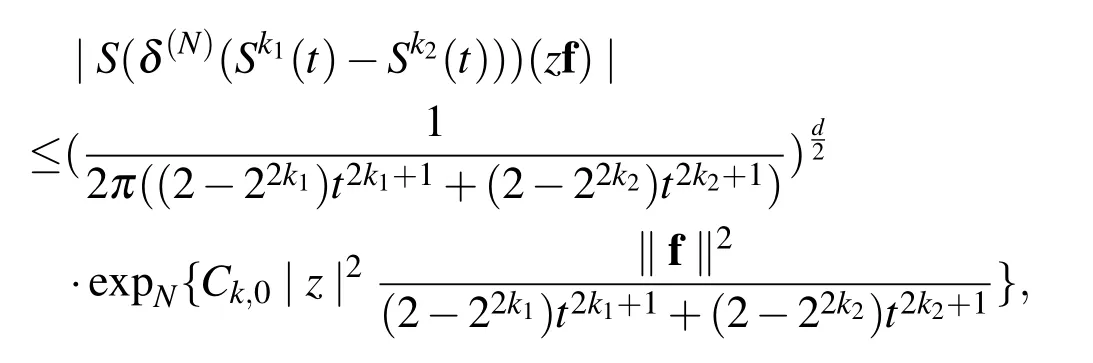
estimating the function expNby

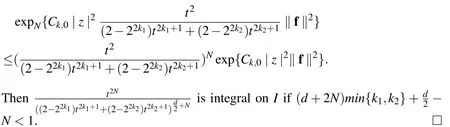
Theorem 3.5.For each k1,,every positive integer d≥1and N≥0satisfied(d+2N)min,the truncated local timesconvergesstrongly to truncated local timesinwhenεtends to zero.

proof:By S-transform ofgiven by

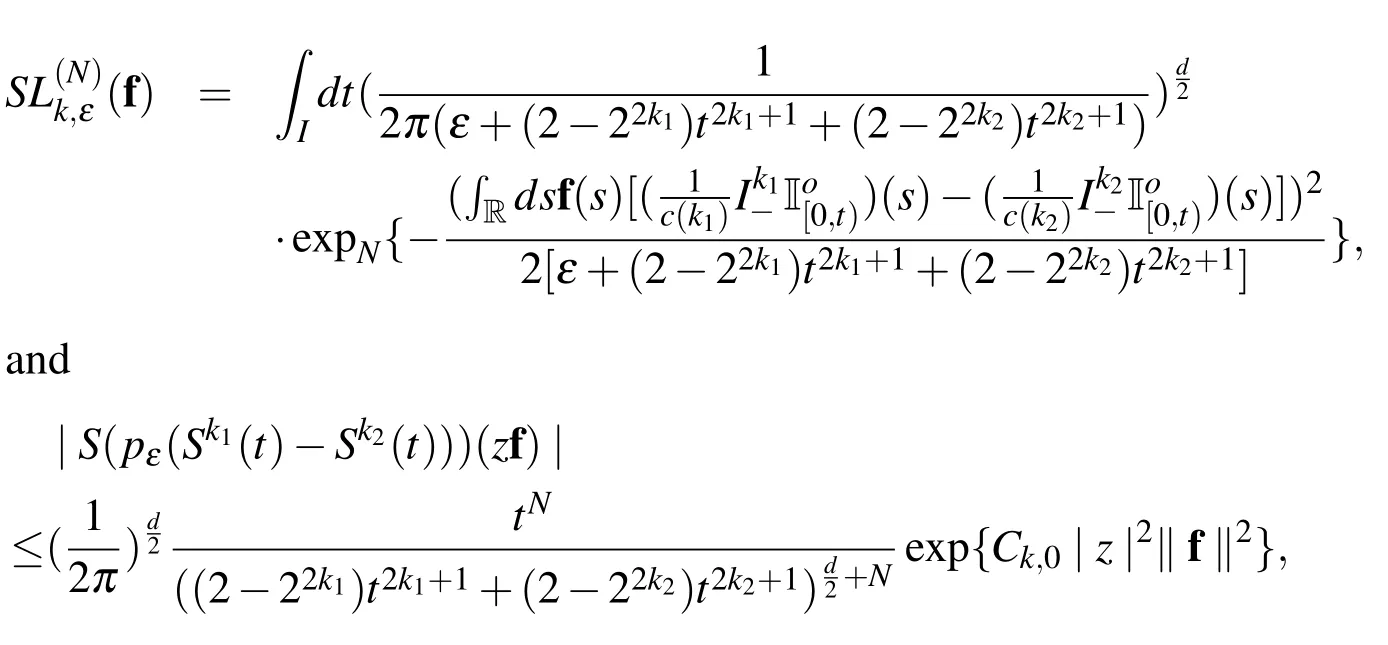
On the other hand,there is

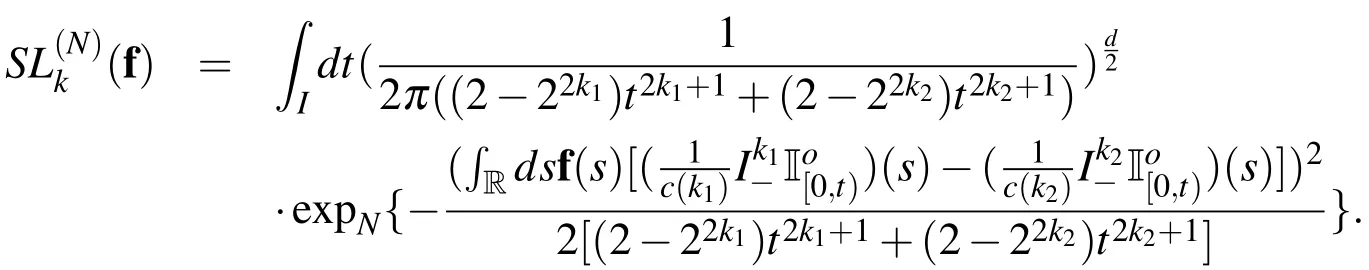
It follows from the above inequality withz=1 and by a dominated criterion,the(f)converges towhenεtends to zero.Applying Lemma 2.2,we obtain the required convergence.

To study the existence of local time of sfBms in(L2),we need verify the following lemmas.

Lemma 3.6.For each uis a decreasing function ofkwhere

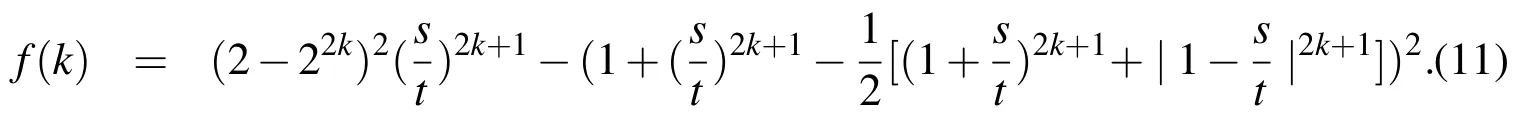
proof:Making change variance(11)can be written as

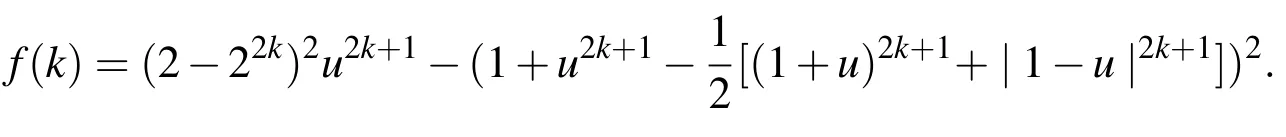
We discuss the properties off(k).The derivative off(k)is given by

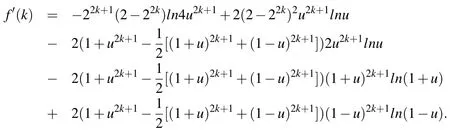
Introduce the following notations

Now we show that the first part is negative.In fact

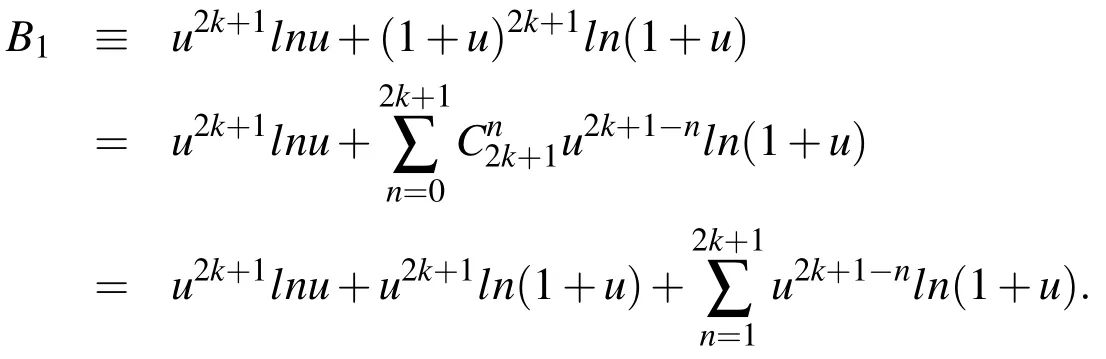
Notice thaty=lnuis an increasing function,and there exist

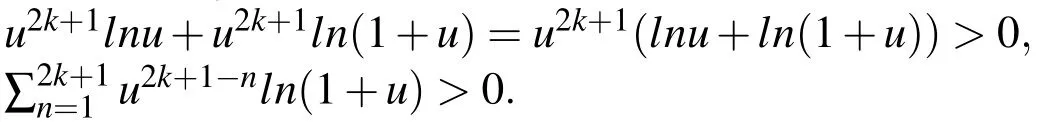
ThereforeB1＞0.Foruwegetu＞1-u.Forα≡2k+1∈(0,2),y=uαisalso an increasing function.Then

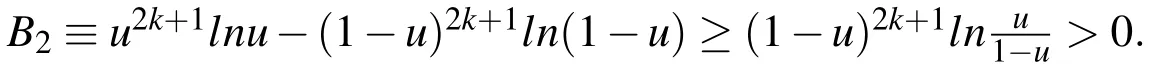
Note thatBis negative.Thusf′(k)＜0,which means thatf(k)is a decreasing function of

Lemma 3.7.For each kandε＞0,there exists0＜△5＜1,where

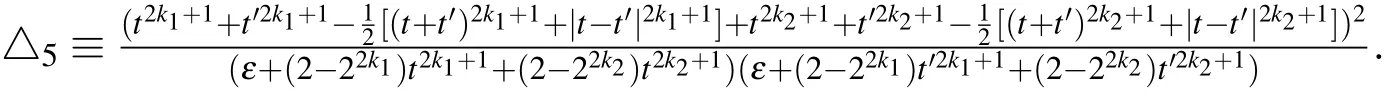
proof:Fork,according to results in[Bojdecki(2004)],there isCh(s,t)＜Rh(s,t),whereCh(s,t)andRh(s,t)denote the covarianceof sfBm and fBm,respectively.We obtain

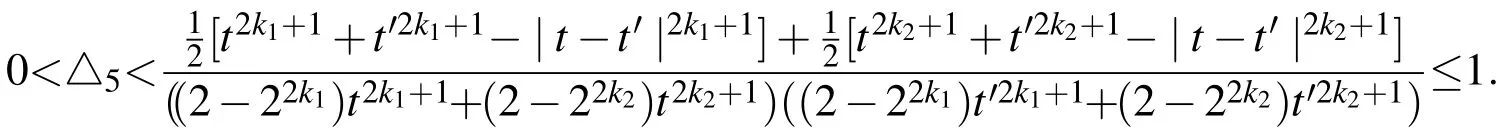
On theotherhand,forkwe rew rite the△5as follows

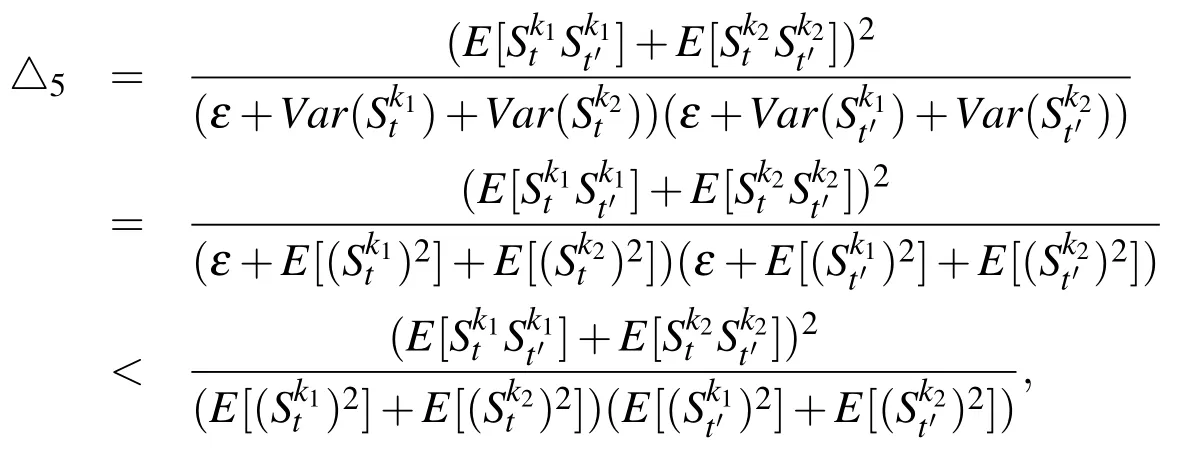
for∀ε＞0.To finish the proof,we need verify

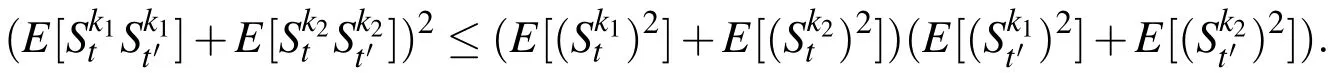
In fact,the last inequality above is tested by following fact(xy+zy)2≤(x2+z2)(y2+h2),forx,y,z,h

Lemma3.6 and Lemma3.7 imply the following theorem

Theorem 3.8.Forany pairofk1,k2and every positive integerd≥1suchthatd＜,Lk,εconverges to Lk in(L2)asεtends to zero.

proof:By Theorem 3.3 and Proposition 3.4,we consider chaos expansion of.We need show that the sums

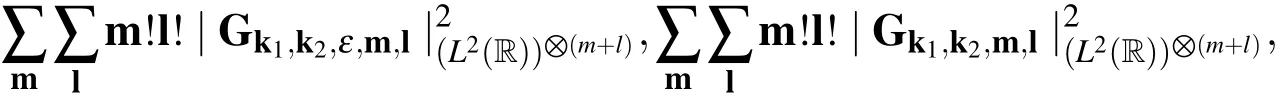
converge,whenεtends to zero.

Step 1.Letus consider the convergence of the firstsum in(L2).

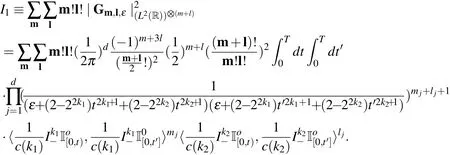
Fori=1,2,there is

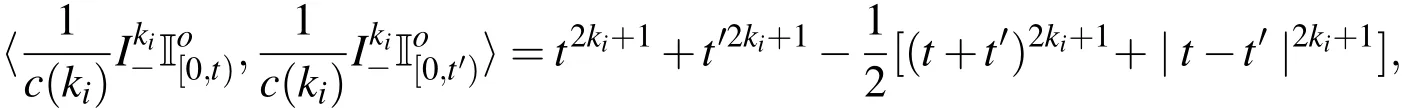
Hence

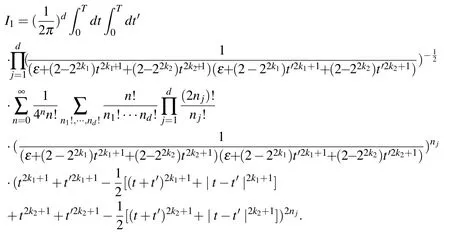
As similar as the proof of Theorem 12 in[Oliveira et.al(2011)]and by Lemma 3.7,the integrand ofI1can be rew ritten as

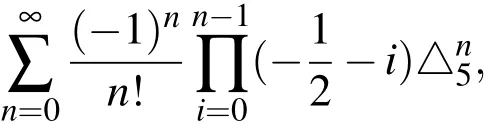
Comparing with the Taylorexpansion of the function,I1becomes

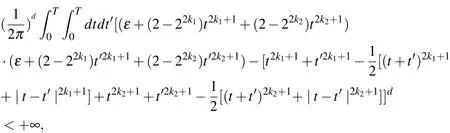
Step 2.Letus consider the convergence of the second sum in(L2).

Similarly,we takeε=0 and obtainequal toAs a result,we obtain

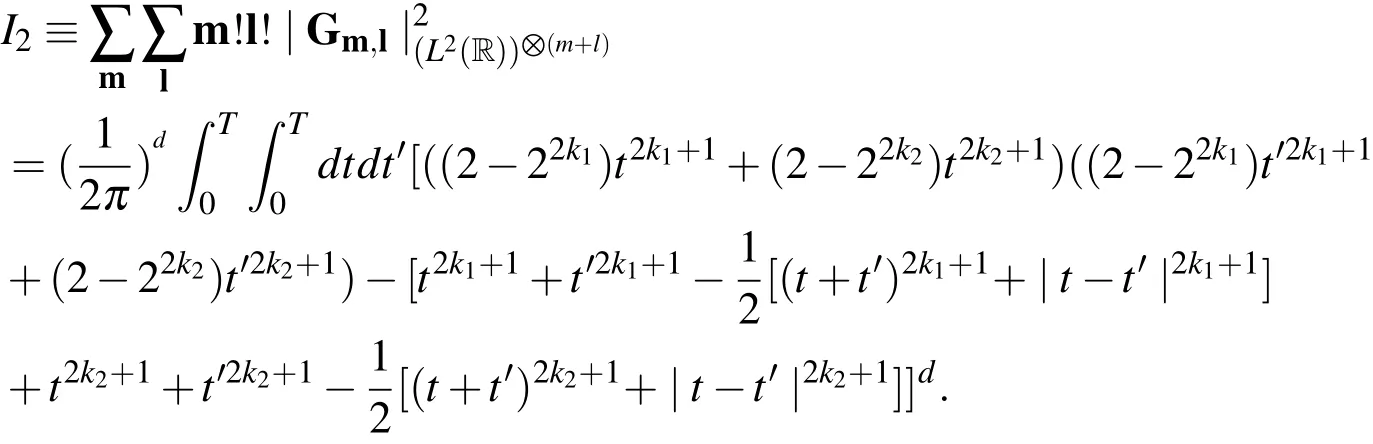
When replaceδfunction with Gaussian sequence,similar techniques in[Nualart et.al(2007);Oliveiraet.al(2011)]allow us to consider singular points.

It is easily to see that

Denote

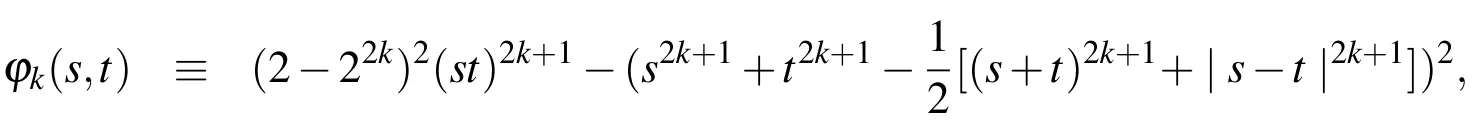
which is a homogeneous function with respect tosandtwith theorder4k+2.For 0＜s＜t＜T,

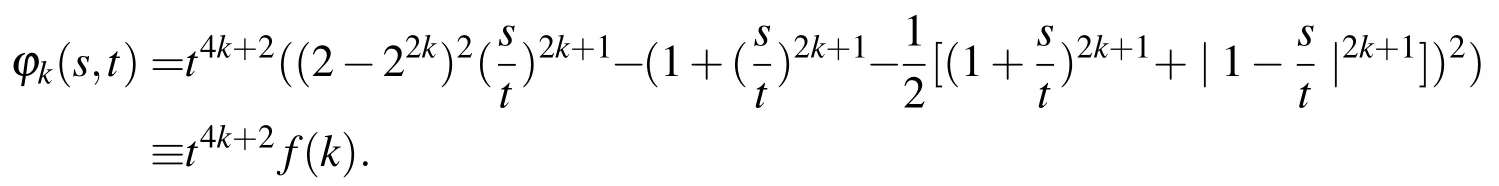
Using Fubini’s theorem and the fact

and takingT=1 for simplicity,the multiple integral inI2becomes

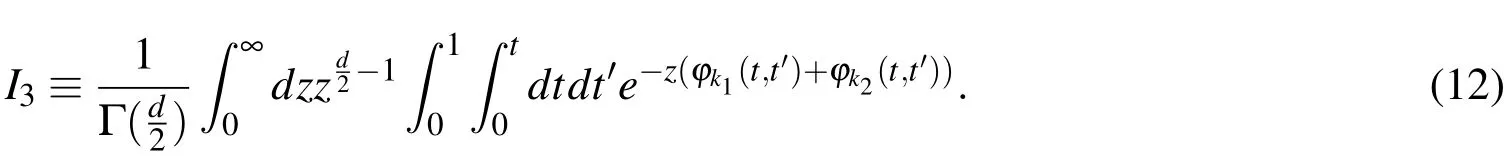
As forallz∈[0,1],the integral in(12)is convergentin aneighborhood of zero.By Lemma3.6,we get the following fact

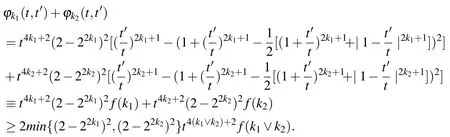
Therefore

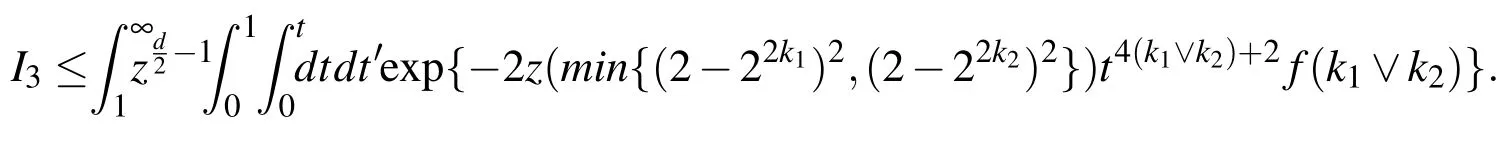
Comparing with the homogeneity properties of

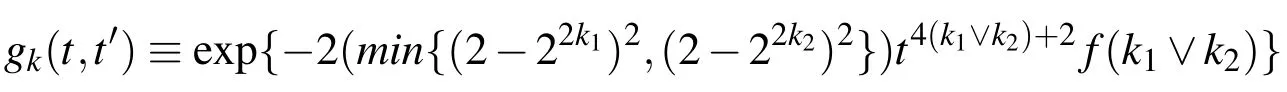
with respect totandt′,the furtherest imation is obtained

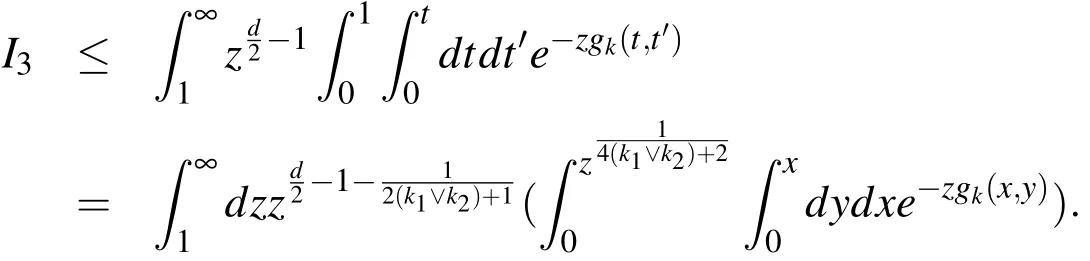
Using that{(x,y):and making a polar change of coordinates,weget

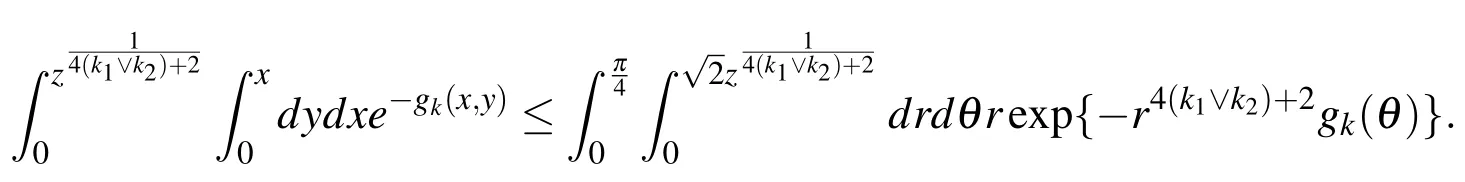
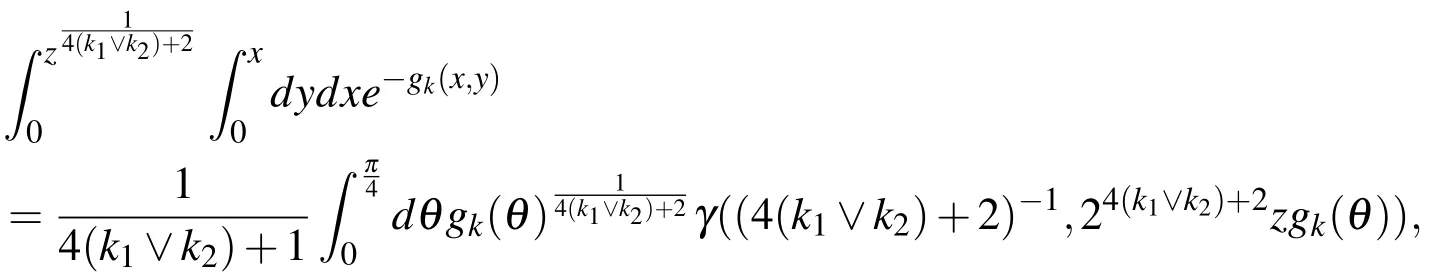
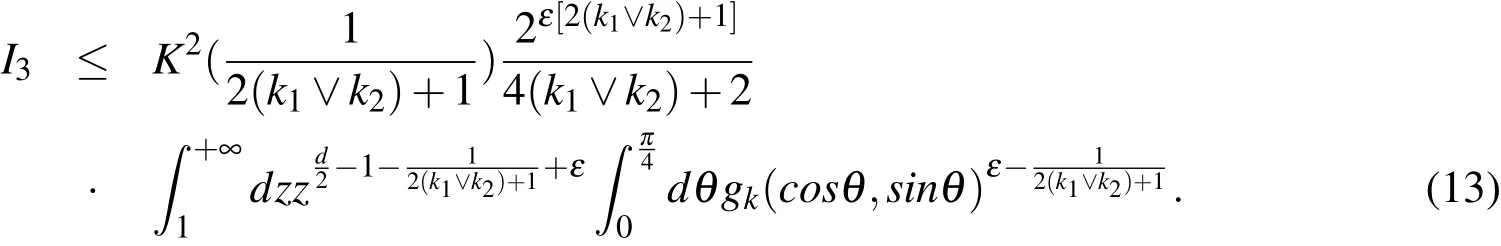
The integral inzconverges whenAnother integral with respect toθis also convergence.Thus(13)is convergence.

Acknowledgement:The project is supported by the National Natural Science Foundation of China(No.71561017),Natural Science Foundation of Gansu Province(No.145RJZA033),Foundation of Research Center of Quantitative Analysis of Gansu Economic Development(No.SLYB201202).

Bender,C.(2003):An Itôformula for generalized of a fractional Brownian motions with arbitrary Hurst para meter.Stoc.Proces.Appl.,vol.104,pp.81-106.

Biagin,F.;Hu,Y.Z.;Φksendal B.;Zhang,T.S.(2008):Stochastic calculus for fractional Brownian motion and applications.London:Springer-Verlag.

Bojdecki,T.;Gorostiza,L.;Talarczyk,A.(2004):Sub-fractional Brownian motion and its relation to occupation times.Statis.Proba.Letters,vol.69,pp.405-419.

Chen,Y.;Han,X.;Liu,L.(2014):Numerical solution for a class of linear system of fractional differential equations by the Haar wavelet method and the convergence analysis.Compu.Model.Engin.Scien.,vol.97,No.5,pp.391-405.

Drumond,C.;O liveira,M.;Silva,J.(2008):Intersection local times of fractional Brownian motions withH∈(0,1)as generalized whit enoise functionals.5th Jagna Inte.Workshop Stoch.Quan.Dyna.Biom.Syst.,vol.1021,pp.34-45.

Hu,Y.;Nualart,D.(2007):Regularity of renormalized self-intersection local time for fractional Brownian motion.Commun.Inf.Syst,vol.7,pp.21-30.

Jiang,Y.;Wang,Y.(2007):On the collision local time of fractional Brownian motions.Chin.Ann.Math.,vol.28,pp.311-320.

Liu,J.;Peng,Z.;Tang,D.;Cang,Y.(2012):On the self-intersection local time of subfractional Brownianmotion.Abstr.Appl.Analy.,vol.2012,pp.1-27.

Nualart,D.;Ortiz-Latorre,S.(2007):Intersection local time for two independent fractional Brownianmotions.J.Theor.Prob.,vol.20,pp.759-767.

O liveira,M.;Silva,J.;Streit,L.(2011):Intersection local times of independent fractional Brownian motions as generalized white noise functionals.Acta Appl.Math.,vol.113,pp.17-39.

Tudor,C.(2008):Inner product spaces of integrands associated to subfractional Brownian motion.Statis.Proba.Letters,vol.78,pp.2201-2209.

Yan,L.;Shen,G.(2010):On the collision local time of sub-fractional Brownian motions.Statis.Proba.Letters,vol.80,pp.296-308.

Wang,J.;Liu,L.;Chen,Y.;et.al(2015):Numerical study for a class of variable order fractional integral-differential equation in terms of Bernstein polynom ials.Compu.Model.Engin.Scien.,vol.104,No.1,pp.69-85.

Wang,X.J.;Guo,J.J.;Jiang,G.(2011):Collision local times of two independent fractional Brownian motions.Front.Mathe.in China,vol.6,pp.325-338.