Effcient hybrid method for time reversal superresolution imaging
Xiaohua Wang,Wei Gao,and Bingzhong Wang
School of Physical Electronics,University of Electronic Science and Technology of China,Chengdu 610054,China
Effcient hybrid method for time reversal superresolution imaging
Xiaohua Wang*,Wei Gao,and Bingzhong Wang
School of Physical Electronics,University of Electronic Science and Technology of China,Chengdu 610054,China
An effcient hybrid time reversal(TR)imaging method based on signal subspace and noise subspace is proposed for electromagnetic superresolution detecting and imaging.First,the locations of targets are estimated by the transmitting-mode decomposition of the TR operator(DORT)method employing the signal subspace.Then,the TR multiple signal classifcation(TR-MUSIC) method employing the noise subspace is used in the estimated target area to get the superresolution imaging of targets.Two examples with homogeneous and inhomogeneous background mediums are considered,respectively.The results show that the proposed hybrid method has advantages in CPU time and memory cost because of the combination of rough and fne imaging.
time reversal(TR),decomposition of the time-reversal operator(DORT)method,multiple signal classifcation(MUSIC) method,superresolution,imaging.
1.Introduction
The time reversal(TR)technique has attracted a lot of attention for its spatial-temporal focusing characteristics in domains such as imaging,communication,location, and nondestructive evaluation.In the imaging domain,generally,TR imaging techniques can be divided into two categories:wave back propagation methods and TR operator methods.The TR mirror method(TRM)[1,2]and the iterative TR method(ITRM)[3,4]are based on wave back propagation.However,they cannot focus on weaker targets in the situation of multiple objects because of the spatial focusing characteristics of TR electromagnetic waves.To overcome this fatal faw,some improved methods based on the decomposition of the TR operator(TRO)are developed,such as[5,6].These methods,defned by the multistatic response(MSR)matrix,can be classifed as the decomposition of the time reversal operator(DORT)methods[7–10]and time reversal multiple signal classifcation(TR-MUSIC)methods[11–13].Among these methods,the transmitting-mode electromagnetic TR-MUSIC method was investigated for surveillance in wireless sensor network(WSN)by employing the pseudo-spectrum deduced from the Maxwell equations[13].Compared with the echo-mode TRO imaging methods,the transmittingmode TRO methods can get more accurate locations and higher resolutions.Additionally,the performances of TRO-based imaging techniques under clutter or additive noise were investigated in[12–14].
Throughout the existing imaging methods based on the TR technique,time domain methods have the properties of statistical stability[15],but they cost more computing resource.The central frequency TR-MUSIC method could get the superresolution results without considering the effciency[14],but this method cannot provide a stable image of the point-like targets under high-level clutter and noise.The DORT method is more robust than the TRMUSIC method under high-level clutter or noise,but it can only give low resolution results.
Therefore,the effciency and memory cost of the TRO-based superresolution imaging method will be concentrated on.An effcient hybrid TRO-based imaging method will be proposed,which is especially suitable for the targets whose sizes and separation are less than the diffraction limit.Under the assumption of transmitting-mode,the DORT method using signal subspace is employed to provide a primary estimation of the target area.Then,the TRMUSIC method using noise subspace is employed to provide superresolution imaging of the targets.
2.Hybrid TR imaging method
Suppose the analytical model is composed of a transmitter array Tx(located at αt),M small anisotropic spheres as the targets(located at mi,i=1,2,...,M),and a receiver array Rx(located at αr).In addition,the transmitter andthe receiver arrays are bistatically and randomly located in space.And each element of the transceiver/receiver arrays consists of three dipoles oriented in the x,y,and z directions,respectively.If the source of the transmitter array element at αtis J(αt),the incident electromagneticfelds at the targets can be expressed as follows:
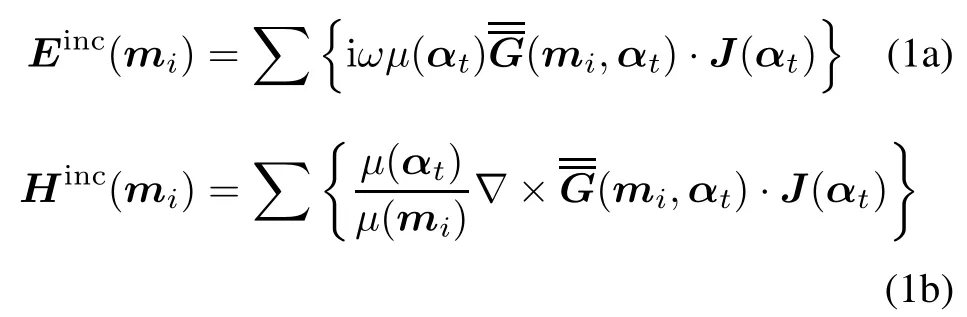
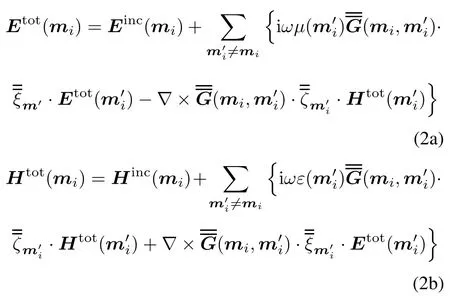
where ε is the permittivity of the background,andare the electric and the magnetic polarization strength tensors of the targetrespectively.Therefore,the scattering electric felds at the receiver array can be expressed as

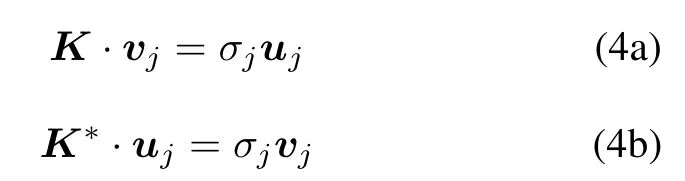
Therefore,the voltages induced on the antenna dipoles can be obtained.Then,according to the relation of voltages and currents,the MSR matrix,K,is gotten.And the TRO can be expressed as T=K∗K.More details can be found in[13].Based on the singular value decomposition of matrix K,the following equations can be gotten: where the operation“∗”denotes the conjugate transpose, σjis the jth singular value,and ujand vjdenote the jth column singular vectors of the unitary matrices U and V,respectively.Both of the matrices U and V can be divided into signal subspace and noise subspace,which are spanned by the singular vectors corresponding to the nonzero and zero singular values,respectively.Therefore, the corresponding imaging pseudo-spectrums,PDORT(r) of the transmitting-mode DORT method employing signal subspace and PTR-MUSIC(r)of the transmitting-mode TR-MUSIC method employing noise subspace,can be expressed as
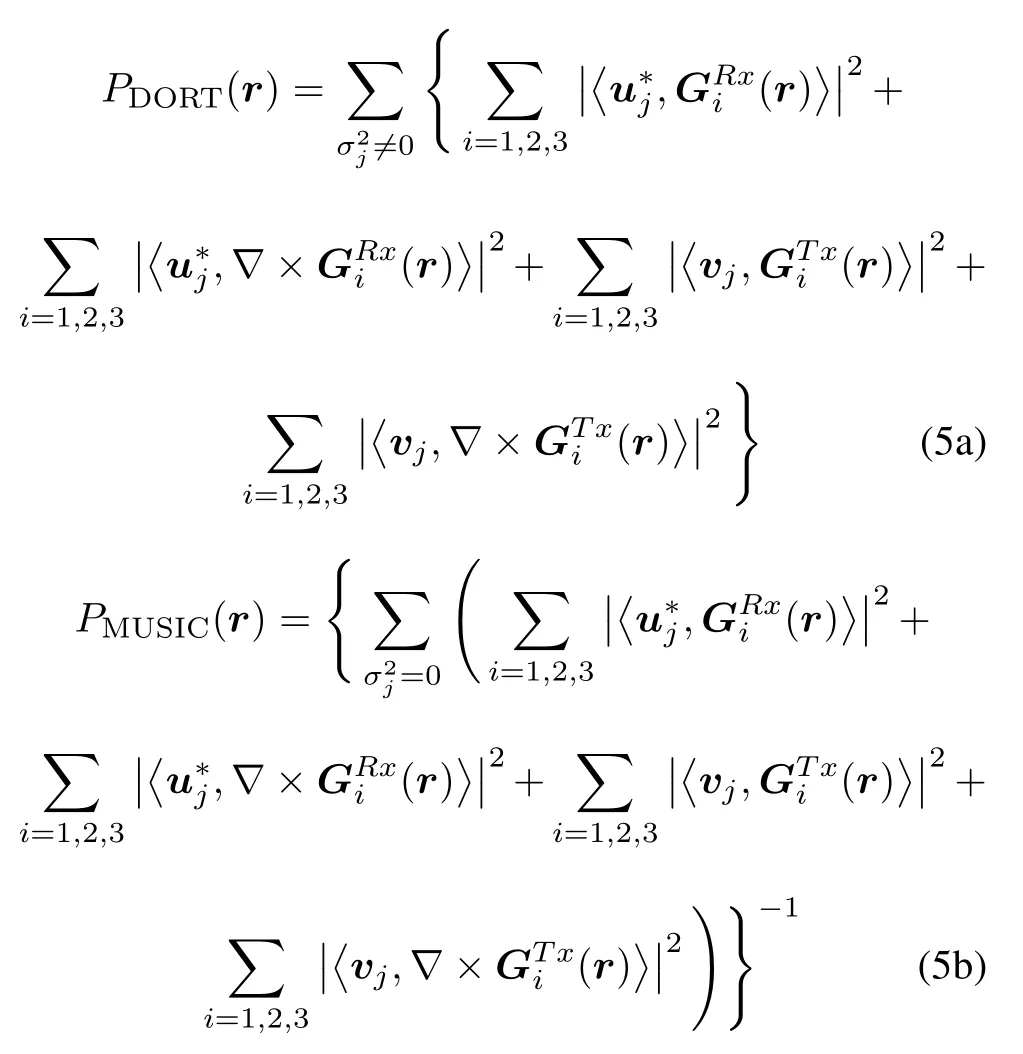
where GRx(r)and GTx(r)are the Green’s function vectors between the scanning point and Tx,Rx,respectively.It is obvious that the similarity of the two methods is they are based on the same singular value decomposition, and the main difference is they employ different subspaces.
Throughout the existing works,compared with the TRMUSIC method,the advantage of the DORT method is more stable under high-level clutter or noise scenario.Meanwhile,the advantages of the TR-MUISC method are:(i)when the targets are not well-resolved in space,the TR-MUSIC method can still work while the DORT method may be invalid;and(ii)the TR-MUSIC method can overcome the diffraction limitation to get a superresolution imaging of the targets.Obviously,the advantages of the DORT method are the disadvantages of the TR-MUSIC method or vice versa.Therefore,for well using the advantages of the two methods,an effcient and stable hybrid imaging method with superresolution can be proposed by taking the following procedures:
Step 1Employing the DORT method in the whole imaging domain with lower resolution to get a primary estimation of the targets with little CPU time and memory cost.
Step 2Employing the TR-MUSIC method in the target area estimated by the DORT method to get a superresolution image of the targets beyond the diffraction limitation.
Since the imaging domain by Step 2 is much smaller than the original imaging domain,much more computing time and memory will be saved compared with those employing the TR-MUSIC method in the whole imaging domain with the same resolution.Additionally,the resolution in Step 1 and Step 2 should be fexible to get a tradeoff between the effciency and the resolution in practice.
3.Numerical validation
To verify the effciency and stability of the proposed hybrid method,two examples are considered in this paper.The frst one is for the detection in WSN(homogeneous background medium)with free space dyadic Green’s functions, and the second one is for the underground detection(inhomogeneous background medium)with subspace dyadic Green’s functions.Details of the dyadic Green’s functions can be found in[13,16].In the two simulations,multiple scattering is considered.In addition,the additive white Gaussian noise is added to contaminate the MSR matrix, K,to realize a certain level of signal to noise ratio(SNR) as in[13].In this paper,the resolution is in proportion to the wavelength,and both of the operation frequencies in the two simulations are set as 300 MHz for simplicity.All the imaging pseudo-spectra are normalized as dB.The calculations are carried out on a workstation with two Intel Xeon E5-2640CPUs and 196 GB RAM,and the programs are compiled by Matlab software(version 7.11)without parallel processing.
3.1Example with homogeneous background medium in WSN detection
In this simulation,the confguration of the TR imaging model is shown in Fig.1(a)with homogeneous background medium.32 transmitter antenna elements and 32 receiver antenna elements are employed.The transmitter and the receiver antenna arrays are planar distribution and 10λ away from the center of the imaging domain.For getting an effective array aperture,the spacing between the elements is set to 4λ in this example.Two anisotropic targets with a radius of λ/30 are placed at points(3λ,3λ,3λ)and(3.4λ, 3λ,3λ),respectively,in the three dimensional imaging domain of“6λ×6λ×6λ”.The principal elements of the permittivity and permeability tensors and the Euler rotational angels are set as the same as those in[13].


Fig.1 Three-dimension transmitting-mode imaging when SNR= 40 dB
When SNR=40 dB,Fig.1(b)gives the normalized singular values of the MSR matrix,and the prior 12 column singular vectors,whose corresponding singular values are greater than zero,belong to the so-called signal subspace and the rest belong to the noise subspace.Figs.1(c)and 1(d)show the imaging results of the transmitting-mode TR-MUSIC and DORT methods when the resolution is set as λ/4,respectively.It can be seen that the TR-MUSIC method gives a higher resolution while the DORT method cannot give a good resolution result for such non-well separated targets as expected.But from Fig.1(d),the target area can be distinguished as the marked black cube.Therefore,for getting a better resolution imaging results,the TRMUSIC method can then be further used alone in this estimated target area.
Fig.1(e)gives the last results with λ/20 superresolution.From this fgure,the number of targets can easily be gotten.Fig.2 depicts the CPU time and memory cost by taking the DORT method,the TR-MUSIC method and the proposed hybrid method.It can be seen that the hybrid method has great advantages in both CPU time and memory cost owing to the strategy in Section 2.
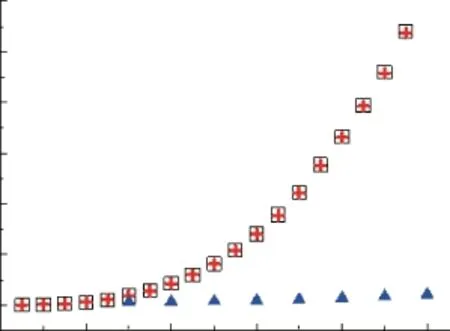

Fig.2 Comparisons of three methods
3.2Example with homogeneous background medium in WSN detection
In the second simulation,the confguration of the underground detection model is shown in Fig.3(a).The ground plane is set at the z=0 plane.And when z<0,the corresponding space is underground.The permittivity is set as ε+=ε0for the air space and ε−=4.5ε0for the underground,while the permeability is μ0in the whole space.In this example,36 transmitter antenna elements and 36 receiver antenna elements are used.The two planar antenna arrays are placed in the z=2λ plane and separated with a distance of 4λ.The properties of the targets are the same as those in the frst example.Two anisotropic spheres are placed at points(5λ,5λ,−3λ)and(5.4λ,5λ,−3λ),respectively,in the three dimensional imaging domain of“10λ×10λ×5λ”,separated 0.4λ away from each other.The imaging domain is one wavelength away from the ground plane.
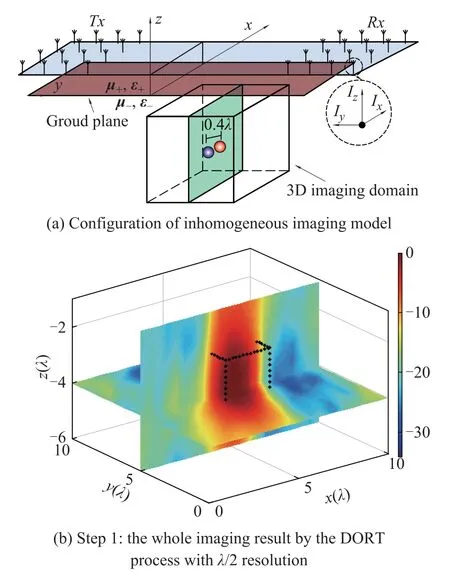
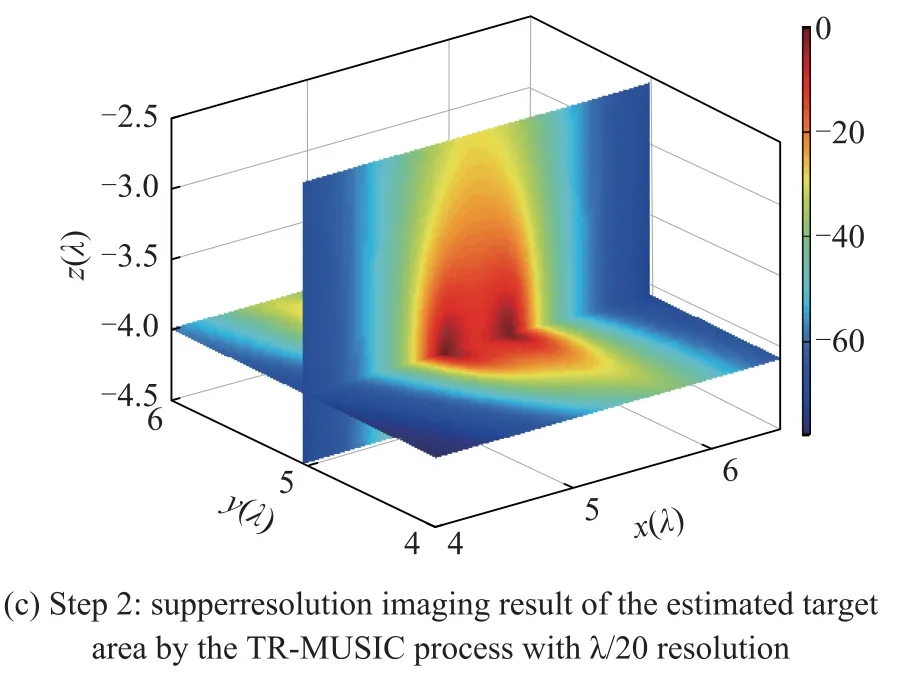
Fig.3 Imaging results when SNR=80 dB
Fig.3(b)shows the result of the frst DORT process when the resolution is set as λ/2.Then,according to the estimated target area,a stable superresolution imaging of two targets in a subwavelength scale is shown in Fig.3(c) by taking the TR-MUSIC process with a resolution of λ/20.Fig.4 gives the superresolution imaging results by the proposed method in different SNRs.It can be seen that the additive noise and clutter have a great infuence to the TR imaging.Thus,in practical application,to get a good superresolution imaging,a high SNR condition is necessary.

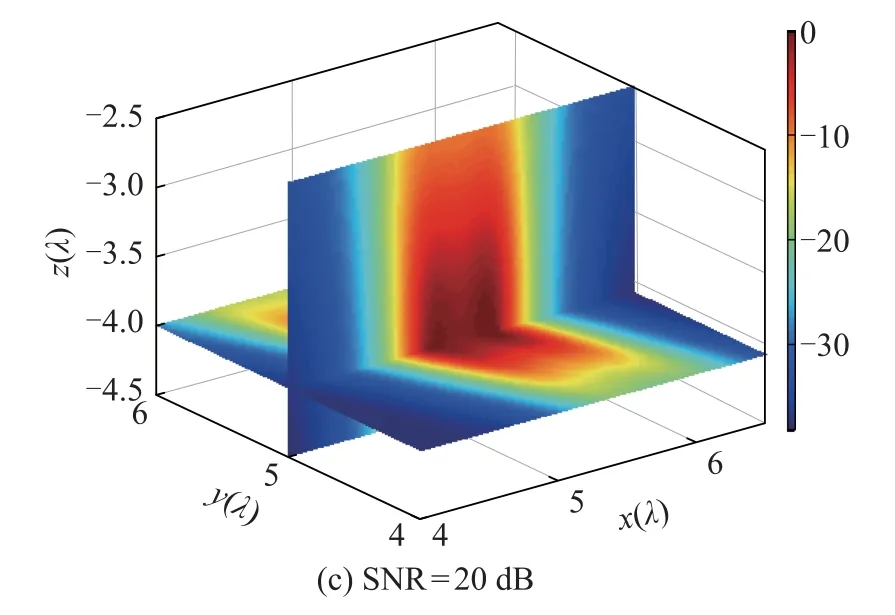
Fig.4 Supperresolution imaging results with different SNRs in the target area
4.Conclusion and discussion
On the framework of transmitting-mode electromagnetic TR imaging,a hybrid DORT and TR-MUSIC method is proposed for the fast superresolution TR imaging and detection.The proposed method employs the low resolution DORT method to get the primary estimated target area at frst,and then employs the high resolution TR-MUISIC method to get superresolution imaging.Two simulations show that the proposed strategy costs less computing time and memory than those by the DORT or TR-MUSIC methods.The new method is a good candidate for complex environment fast superresolution imaging and detection.Although in this work,the confgurations of the TR imaging technique are based on the transmitting-mode Maxwell form,the idea can be easily extended to other TR imaging models in acoustics and optics.
[1]M.Fink,C.Prada.Acoustic time-reversal mirrors.Inverse Problems,2001,17(2):R1–R38.
[2]J.de Rosny,G.Lerosey,M.Fink.Theory of electromagnetic time-reversal mirrors.IEEE Trans.on Antennas Propagation, 2010,58(10):3139–3149.
[3]C.Prada,J.L.Thomas,M.Fink.The iterative time reversal process:analysis of the convergence.Journal of the Acoustical Society of America,1995,97(1):62–71.
[4]H.C.Song,W.A.Kuperman,W.S.Hodgkiss.Iterative time reversal in the ocean.Journal of the Acoustical Society of America,1999,105(6):3176–3184.
[5]C.Prada,M.Fink.Eigenmodes of the time reversal operator: A solution to selective focusing in multiple-target media.Wave Motion,1994,20(2):151–163.
[6]D.H.Chambers,J.G.Berryman.Analysis of the time-reversal operator for a small spherical scatterer in an electromagnetic feld.IEEE Trans.on Antennas Propagation,2004,52(7): 1729–1738.
[7]M.Davy,J.G.Minonzio,J.de Rosny,et al.Experimental study of the invariants of the time-reversal operator for a dielectric cylinder using separate transmit and receive arrays.IEEE Trans.on Antennas Propagation,2010,58(4):1349–1356.
[8]M.E.Yavuz,F.L.Teixeira.Full time-domain DORT for ultrawideband electromagnetic felds in dispersive,random inhomogeneous media.IEEE Trans.on Antennas Propagation, 2006,54(8):2305–2315.
[9]G.Micolau,M.Saillard,P.Borderies.DORT method as applied to ultrawideband signals for detection of buried objects.IEEE Trans.on Geoscience and Remote Sensing,2003, 41(8):1813–1820.
[10]C.Prada,S.Manneville,D.Spoliansky,et al.Decomposition of the time reversal operator:detection and selective focusing on two scatterers.Journal of the Acoustical Society of America,1996,99(4):2067–2076.
[11]A.J.Devaney.Time reversal imaging of obscured targets from multistatic data.IEEE Trans.on Antennas Propagation,2005, 53(5):1600–1610.
[12]A.J.Devaney,E.A.Marengo,F.K.Gruber.Time-reversalbased imaging and inverse scattering of multiply scattering point targets.Journal of the Acoustical Society of America, 2005,118(5):3129–3138.
[13]X.F.Liu,B.Z.Wang,J.L.W.Li.Transmitting-mode time reversal imaging using music algorithm for surveillance in wireless sensor network.IEEE Trans.on Antennas Propagation, 2012,60(1):220–230.
[14]M.E.Yavuz,F.L.Teixeira.On the sensitivity of time-reversal imaging techniques to model perturbations.IEEE Trans.on Antennas Propagation,2008,56(3):834–843.
[15]J.G.Berryman,L.Borcea,G.C.Papanicolaou,et al.Statistically stable ultrasonic imaging in random media.Journal of the Acoustical Society of America,2002,112(4):1509–1522.
[16]E.Iakovleva,S.Gdoura,D.Lesselier,et al.Multistatic response matrix of a 3-D inclusion in half space and MUSIC imaging.IEEE Trans.on Antennas Propagation,2007,55(9): 2598–2609.
Biographies

Xiaohua Wang was born in 1980.He received his B.S.,M.S.,and Ph.D.degrees from University of Electronic Science and Technology of China(UESTC)in 2002,2005,and 2008,respectively.From March 2008 to February 2009,he was an RF research engineer in Huawei Company.From March 2009 to February 2010,he was with the Department of Electronic Engineering, City University of Hong Kong as research staff.Now,he is an associate professor at UESTC.His research interests include computational electromagnetics,numerical modeling and simulation of the electromagnetic behavior in high-speed integrated circuits and electronic packages, and microwave circuits.
E-mail:xhwang@uestc.edu.cn

Wei Gao was born in 1988.He received his B.S. degree from University of Electronic Science and Technology of China(UESTC)in 2011.He is now pursuing his M.S.degree.His research interests include computational electromagnetics,numerical modeling and simulation of the electromagnetics, and superresolution imaging method.
E-mail:gaoweiuestc@gmail.com

Bingzhong Wang was born in 1962.He received his B.S.,M.Sc.,and Ph.D.degrees from University of Electronic Science and Technology of China(UESTC),Chengdu,China,in 1982,1984, and 1988,respectively,all in electrical engineering.Now,he is a professor of the Institute of Applied Physics,UESTC.Hiscurrent research interests include computational electromagnetics,numerical modeling and simulation of the electromagnetic behavior in high-speed integrated circuits and electronic packages,EMC analysis,electromagnetic modeling by artifcial neural networks,computer-aided design for passive microwave and millimeter wave integrated circuits,and antenna design.
E-mail:bzwang@uestc.edu.cn
10.1109/JSEE.2015.00005
Manuscript received March 17,2014.
*Corresponding author.
This work was supported by the National Natural Science Foundation of China(61301271;61331007),the Specialized Research Fund for the Doctoral Program of Higher Education of China(20110185120008; 20120185130001),and the Fundamental Research Funds for Central Universities(ZYGX2012J043).
 Journal of Systems Engineering and Electronics2015年1期
Journal of Systems Engineering and Electronics2015年1期
- Journal of Systems Engineering and Electronics的其它文章
- Dynamic channel reservation scheme based on priorities in LEO satellite systems
- Adaptive beamforming and phase bias compensation for GNSS receiver
- Novel dual-band antenna for multi-mode GNSS applications
- Nonparametric TOA estimators for low-resolution IR-UWB digital receiver
- Adaptive detection in the presence of signal mismatch
- Estimating DOA and polarization with spatially spread loop and dipole pair array
