Elastoplastic response of functionally graded cemented carbidesdue to thermal loading
Huang Ziqian Li Chuanxin
(1Department of Physics, Southeast University, Nanjing 211189, China)(2College of Physics and Electronic Engineering, Guangxi Teachers Education University, Nanning 530001, China)(3College of Mathematics and Physics, Jingchu University of Technology, Jingmen 448000, China)
One of the latest developments in the field of hardmetals and cements used for mineral cutting and rock drilling operations is the establishment of a functional gradient in near-surface areas of cemented carbide tools leading to functionally graded cemented carbides (FGCC)[1-3]. Advances in materials processing have now provided a variety of technologically viable methods to produce functionally graded materials (FGMs). Such techniques include chemical vapor deposition, physical vapor deposition, powder metallurgy, thermal spray, and self-propagating high-temperature combustion synthesis[4-6]. The evolution of thermal stresses and the associated mechanical responses are influenced by both the geometry of the component and the gradients in the composition and properties.
The elastic constitutive equation and application are available in Ref.[7]. The purpose of this paper is to develop the elastoplastic constitutive response of two-dimensional gradient specimens. The cobalt gradient structure is formed by carburization. The constitutive response for continuous and smooth variation of material properties is developed by constraint factors. Material properties are smooth functions of the volume fraction as a smooth function of position. The field quantities of calculated results change with the function of the cobalt gradient and the temperature difference.
1 Numerical Formulation and Material Model
As mentioned above, a model for continuous and smooth spatial variations in the composition and properties of materials is used. The model is available in the MSC.Marc2005 software.
We consider the small strain problem of axisymmetric solid cylinders. In the solid cylinder, there is a two-dimensional gradient of composition in axial and radial directions. Uniform temperature cooling and heating are assumed. The geometry represents the most realistic geometrical conditions in hard metal tools such as anvils and gradient buttons. The geometry is shown in Fig.1.

Fig.1 Axisymmetric solid cylinder model
In the axisymmetric calculations, a cylindrical coordinate system (r,θ,z) is introduced and all the field quantities are taken to be independent ofθ. The cylinder analyzed numerically is 0≤r≤Rand 0≤z≤L, for which theL/Rratio is 1. Free boundary conditions are assumed on the external boundary of the cylinder,
Tr=Tz=0z=L,r=R
uz=0z=0;ur=0r=0
whereTris the external force in the direction ofr;uzis the displacement in the direction ofz.
The effective values of the coefficient of thermal expansionαand Poisson’s ratioνof the compositionally graded solid cylinder are computed from a volume fraction-based interpolation[8]. In this way,
α=f1α1+f2α2,ν=f1ν1+f2ν2,f1+f2=1
(1)
wherefiis the volume fraction of materiali(0≤f1,f2≤1), and subscript 1 and 2 refer to cobalt and tungsten carbide, respectively. The elastoplastic constitutive relationship developed by constraint factors for FGCCs incorporates the variation of mechanical properties with temperature[2].
2 Numerical Results
2.1 Thermal stress distribution with two-dimensional gradient
Orthogonal intersection for the axial and radial gradient in the corner of the cylinder is considered. The radius and height of the cylinder are 5 and 10 mm, respectively. The thickness of the cobalt gradient zone is around 1 mm. Uniform temperature cooling and free boundary conditions are assumed. Fig.2 shows the distribution of thermal stresses. From Fig.2(a), it is found that the equivalent Von Mises stress mainly concentrates in the cobalt gradient zone and it is quite small in the core. There is a great stress gradient from the cobalt rich zone to the core. The maximum value of the equivalent Von Mises stress is 241 MPa in the surface zone. There are two peaks of the equivalent Von Mises stress which lies in the cobalt rich zone and the surface zone. The picture also shows that the equivalent Von Mises stress in the corner is smaller than that of the counterparts with the same thickness. From Fig.2(b), it can be seen that there forms compressive stresses in the surface zone and tensile stresses in the cobalt rich zone after a temperature cooling from the initial stress-free temperature of 800 to 0 ℃. The maximum value of the surface compressive stress is 254 MPa. The compressive stresses are beneficial to material lifetime.
2.2 Effects of plastic flow
When the cobalt concentration difference in the specimens is equal to or greater than 0.3, there is a distinct plastic flow in the cobalt rich zone. Plastic deformation has a significant effect on the redistribution of the thermal stress. Furthermore, there are different responses between the temperature cooling and heating for the material properties varied with temperature. Tab.1 shows different calculated results in different cobalt concentration differences (Co) and different thermal loadings (cooling or heating).

Fig.2 Stress distribution. (a) Equivalent Von Mises stress after a temperature cooling from initial stress-free temperature of 800 to 0 ℃; (b) Distribution of axial stress after a temperature cooling from initial stress-free temperature of 800 to 0 ℃
Fig.3(a) shows there is distinct strip shaped plastic flow in the cobalt rich zone of the axial gradient after a temperature drop from the initial stress-free temperature of 800 ℃. Fig.3(b) shows that there is distinct plastic flow in the cobalt rich zone of the axial and radial gradient when the temperature increases from 0 to 800 ℃. The maximum value of the total equivalent plastic flow as cooling down is 0.000 18, but the maximum value as heating up is 0.001 4.
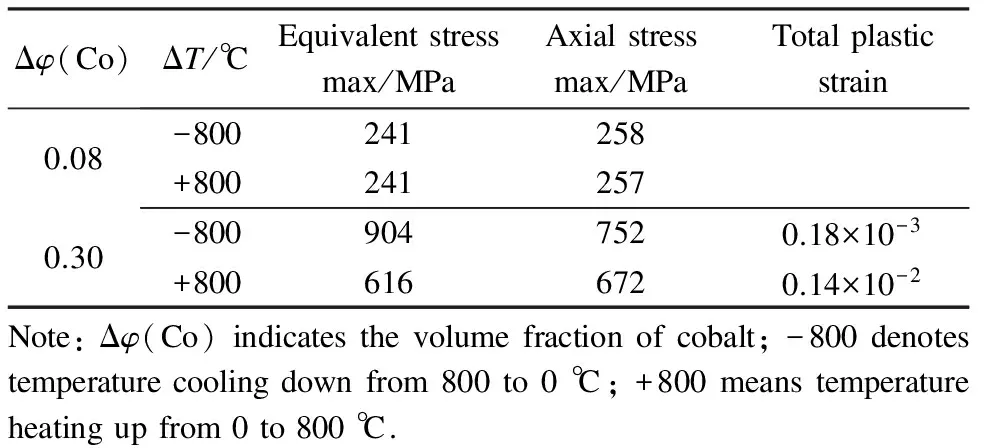
Tab.1 Responses of different cobalt concentration differences and thermal loadings

Fig.3 Distribution of total equivalent plastic strain. (a) After a temperature cooling from 800 to 0 ℃; (b) After a temperature heating from 0 to 800 ℃
Fig.4 shows the effects of the plastic flow on the redistribution of the thermal stress. It can be seen that the difference between elastic and elastoplastic deformation

Fig.4 Distribution of equivalent stress along radial direction in different constitutive relations of materials
after cooling is quite small, but there is a great difference of stress distribution between cooling and heating, which is the same as between elastic and elastoplastic deformation. The difference of the equivalent Von Mises stress between the elastic and the elastoplastic deformation is 20 MPa as temperature cooling and 530 MPa as heating up.
3 Conclusion
Numerical results show the thermal residual stresses mainly concentrate in the cobalt gradient zone and are very small in the core with middle cobalt content. When the temperature drops from the processing, there generate compressive stresses in the surface zone and tensile stresses in the cobalt rich zone. The maximum value of the surface compressive stress is 254 MPa. The compressive stresses are beneficial to material lifetime. When the cobalt concentration difference in the specimens is equal to or greater than 0.3, there is a distinct plastic flow in the cobalt gradient zone. The plastic flow has a significant effect on the reduction of thermal stress.
[1]Lengauer W, Dreyer K. Functionally graded hardmetals[J].JournalofAlloyandCompounds, 2002,338(1/2): 194-212.
[2]Huang Z Q, He Y H, Li P L. Finite element analysis of thermal stresses in functionally graded cemented carbides[J].PowderMetallurgy, 2010,53(4): 278-284.
[3]Narasimhan K, Boppana S P, Bhat D G. Development of a graded TiCN coating for cemented carbide cutting tools-a design approach[J].Wear, 1995,188(1/2): 123-129.
[4]Su Y L, Yao S H, Wei C S, et al. Analysis and design of a WC milling cutter with TiCN coating[J].Wear, 1998,215(1/2): 59-66.
[5]Fan P, Fang Z Z, Guo J. A review of liquid phase migration and methods for fabrication of functionally international graded cemented tungsten carbide[J].InternationalJournalofRefractoryMetalsandHardMaterials, 2013,36: 2-9.
[6]Bull S J, Bhat D G, Staia M H. Properties and performance of commercial TiCN coating. Part I: coating architecture and hardness modeling[J].SurfCoatTech, 2003,163-164: 499-506.
[7]Huang Z Q, He Y H, Cai H T. Finite element analysis of thermal residual stresses at cemented carbide rock drill buttons with cobalt-gradient structure[J].TransNonferrousMetSoc, 2008,18(3): 660-664.
[8]Giannakopoulos A E, Suresh S, Finot M, et al. Elastoplastic analysis of thermal cycling: layered materials with compositional gradients[J].ActaMetallMater, 1995,43(4): 1335-1354.
 Journal of Southeast University(English Edition)2013年4期
Journal of Southeast University(English Edition)2013年4期
- Journal of Southeast University(English Edition)的其它文章
- Effect of aggregation intervalon vehicular traffic flow heteroscedasticity
- Application of neural network merging model in dam deformation analysis
- Mechanical analysis of transmission lines based on linear sliding cable element
- Improvement of pump-probe optical measurement technique using double moving stages
- Improvement of pump-probe optical measurement technique using double moving stages
- Annoyance-type speech emotion detection in working environment
