REVIEW OF SOME RESEARCHES ON NANO- AND SUBMICRON BROWNIAN PARTICLE-LADEN TURBULENT FLOW*
LІN Jian-zhong
China Jiliang University, Hangzhou 310018, China
Department of Mechanics, Zhejiang University, Hangzhou310027, China, E-mail: mecjzlin@public.zju.edu.cn HUANG Li-zhong
Department of Mechanics, Zhejiang University, Hangzhou 310027, China
(Received November 17, 2012, Revised November 26, 2012)
REVIEW OF SOME RESEARCHES ON NANO- AND SUBMICRON BROWNIAN PARTICLE-LADEN TURBULENT FLOW*
LІN Jian-zhong
China Jiliang University, Hangzhou 310018, China
Department of Mechanics, Zhejiang University, Hangzhou310027, China, E-mail: mecjzlin@public.zju.edu.cn HUANG Li-zhong
Department of Mechanics, Zhejiang University, Hangzhou 310027, China
(Received November 17, 2012, Revised November 26, 2012)
The study of nano- and submicron Brownian particle-laden turbulent flow has wide industrial applicability and hence has received much attention. The purpose of the present paper is to provide and review some researches in this field. The topics are related to the universality, particularity, complexity and importance of nano- and submicron Brownian particle-laden turbulent flow, the models of particle general dynamical equation, the collision behavior of particles. Finally, several open research issues are identified.
particle-laden flow, nano-particle, submicron particle, Brownian, turbulence
Introduction
Universality:
Nano- and submicron particle-laden flow is ubiquitous in nature and is widely used in the fields of materials science, engineering thermal physics, chemical industry, light industry, medicine and medical care, food and beverage, refrigeration, and so on.
Atmospheric pollutants are mainly nano- and submicron-particles that come from smoke from industrial chimneys, garbage burning, welding gas and fog, dust storms, and other natural and industrial processes. Particle mass concentrations of gasoline or diesel engine exhaust emissions have been greatly reduced, but the number of nano- and submicron-particles from the formation of nucleation by means of homogeneous condensation has been actually exponential growth which leads to serious environment problems because of high concentration of particle emissions. The motion of pollutant particles in the generation, synthesis, transport, coagulation and condensation process is atypical one of nano- and submicron particle-laden flow.
Іn the synthesis process of nano-particles by way of gas phase combustion and in the nanomaterial manufacturing process via nanoclusters or monomer particles as matrix, the structure, scale, chemical composition of coagulated nano-particles and the degree of dispersion of nano-particles are closely related to the nano-particle-laden flow. The nano- and submicron-particles have large specific surface area, high activity and diffusion rate, so when nanometer powder is sintering, the speed of densification will be high, the sintering temperature can be also reduced and mechanical properties will be improved. When the size of particle is smaller than a certain threshold value, the particle will turn into superparamagnetic state. With the performance of high coercive force and giant magnetoresistance in this state, the material can be used to produce magnetic refrigerator, permanent magnetic material, magnetic fluid, magnetic recording device, magneto-optical element, magnetic storage element and magnetic detector. Magnetic fluid can be used for dynamic sealing, novel lubricant, enhancing power of speaker, damping devices, specific gravity separation, etc., Changing from typical ferroelectric material into para-electric one, metal nano-particles at low temperature will be insulation which can be used to produceconductive paste, insulating paste, electrode, superconductor, quantum device, electrostatic shielding material, pressure sensitive and nonlinear resistance, thermoelectric material, etc., Adding metal nano-particles into chemical fiber products will reduce electrostatic effect. The manufacture of these materials mentioned above involves the nano- and submicron particle-laden flow. Іn the field of heat and mass transfer, it becomes an effective means to increase convective heat transfer coefficient by adding nano-particles into liquid, and the coagulation or deposition of particles in motion will directly influence this increment.
Ailments will happen when particles are sucked into and then deposit in the respiratory tract. Nanoparticles are much easier to enter the lungs and deposit in the alveoli. Moreover, there is high concentration of trace metal elements and organic pollutants on the surface of nano-particles. Therefore, nano-particles do greater harm to human health than conventional pollutant particles. Іn order to solve this problem, it is needed to study convection and transport of nano-particles far away from the deposition surface and diffusion and adsorption of nano-particles near the deposition surface. Transmission of drug at nanometer scale is a new field to study. Only drug particles in a certain size can be transported to the diseased parts. Drug nano-particles will become coagulated fractal structure in the respiratory transmission due to van der Waals forces and Brownian motion that will change their dynamic characteristics. Nano-particles can be used for cell separation, cell staining and can also be utilized to produce special drugs or novel antibody for locally targeted therapy. Nano-particles can be employed to separate a very small amount of fetal cells from the blood sample for accurately determining whether the fetal cells with genetic defects. Cell separation by using nano-particles can identify cancer cells from blood of tumor inchoate, thereby cancer can be diagnosed and treated earlier.
Іt can be seen that nano- and submicron particle-laden flow has a wide range of applications.
Particularity:
Nano- and submicron particle-laden flow has different characteristics from those of micron- and larger-sized particle-laden flows.
The first characteristic is the small-scale effect. Besides the Stokes force generally required to consider, for nano- and submicron-particles, the London-Van Der Waals force, electrostatic force, the fluctuating force caused by Brownian motion, etc. should also be considered. Dispersion effect of surrounding gaseous molecules has a greater impact on the dynamic characteristics of particles. Due to small size of a single particle and large particle number density in the system of nano- and submicron particles, it is generally concerned about the comprehensive effect reflected by large number of particles, such as the number density, mass concentration, scale dispersion, particle mean diameter, variance of particle diameter, and so on.
The second characteristic is the cross-scale effect. The change in a physical quantity under the microscopic mechanism must be embodied in the expression of the macroscopic quantity during research because the motion theory of nano- and submicronparticles is between the molecular kinetic theory and the continuum theory. There is a multi-scale spectrum distribution in the nano- and submicron particle-laden flow. Both large-scale flow field information and small-scale particles information exist in flow fields. There exists significant differences in relaxation time among the evolution of flow field, particle collision and phase transition.
The third characteristic is the existence of phase transition and scale variation. Іn nano- and submicron particle-laden flow, the phase transition and scale variation are evolved in gas-solid or gas-liquid phase transition (i.e., nucleation), particle migration induced by thermophoresis or electric field, coalescence between particles caused by turbulence and Brownian motion, increase of particle volume induced by heterogeneous coagulation or chemical reaction on the surface of particles, particle breakage caused by turbulence of flow field, particle deposition on the wall, etc., Therefore, in nano- and submicron particle-laden flow, special attention must be paid to the evolution of particle surface area concentration, chemical constituents of particle, and fractal dimension of the coagulated particles.
Complexity:
Up to now there is no mature model describing the movement of nano- and submicron-particles because the movement is affected by many factors. And the issue will become very complicated especially when the influence of Brownian motion and fluctuating force from turbulent flow field on particles is equally important. Comparing nano- and submicron particle-laden flow with micron and larger-sized particle-laden flow, one important difference is that new particles will generate for the former with aggregation and coagulation between particles. This is always an integrated chemical and mechanical process.
Nano- and submicron particle-laden flow is usually a multi-field-coupled, multi-component and multiscale flow. Higher-order differential-integral equations are used to describe evolution of particle number density which is usually very high, so it is extremely difficult to numerically simulate such flows. Іn experiments, the significant difference in geometry scale and characteristic time between particles and flow field makes it difficult to investigate.
The influence of flow turbulence on nano- and submicron particle-laden flow is very important. Іt can be seen not only from the diffusion effect of turbule-nce on particle phase, but also from the fact that, in most cases, turbulence dominates the particle nucleation, coalescence, chemical or heterogeneous coagulation, particle breakage etc., Moreover, there are still no appropriate methods to treat crystallized nucleation, coalescence between particles, coagulation and surface growth caused by turbulent fluctuation.
Іmportance:
Researching and understanding the dynamic characteristics of nano- and submicron particle-laden flow are beneficial to the following aspects: (1) making clear and then controling the distribution of engine exhaust gas, atmospheric nuclear pollution, (2) rationally designing the structure of nanomaterial, (3) optimizing the manufacturing technique of magnetic material, catalyst, pottery, filler constituted by deposition of particles, (4) enhancing the effect of heat and mass transfer in engineering application, (5) improving the efficiency of combustion, (6) improveingthe manufacturing quality of particles in the process of medicines, food, beverages and grain, (7) effectively treating diseases by using drugs in the state of particles. Therefore, the research project is of great importance.
1. Research on models of particle general dynamical equation
The study of nano- and submicron particle-laden flow can be attributed to exploring and revealing the nature of dynamical evolution of particle phase as well as their transport characteristics in turbulent condition and the interaction between the carrier phase and the dispersed phase. Due to some unique characteristics existing in the nano- and submicron particleladen flow such as the wide particle size distribution, the coexistence of multi-dynamical mechanisms (coagulation, nucleation, particle formation due to chemical reaction, breakage, and condensation) and the turbulence, and the phase transformation, this kind of particle-laden flow undergoes some temporally unsteady-state and spatially non-equilibrium processes under turbulent flow conditions, and especially the dynamical evolution is significantly influenced by some non-linear, unsteady and strong coupling between phases mechanisms. Although some studies have been conducted in this field[1-6], the studies on the turbulent modulation of carrier phase due to the presence of nano- and submicron-particles, the mechanism of evolution of multiphase at the complex phase transformation and coagulation processes, the construction of governing equation with the consideration of strong coupling between phases, and the mechanism of particle collision under the action of turbulence and Brownian motion are still in their infancy, and thus they need to be further conducted.
Nowadays, the one-way coupling method has been mostly used in the study on the nano- and submicron particle-laden flow within a two-fluid framework[7-9]. Іn this kind of study, when applying the Reynolds averaged approach (RAA) to the governing equation for the disperse phase, i.e., the Particle General Dynamical Equation (PGDE), the turbulent fluctuation only takes effect in the turbulent diffusion term, while its contribution to dynamical processes, including nucleation, particle formation due to chemical reaction, coagulation and surface growth due to condensation, is always ignored. Іn fact, when the RAA is applied to the PGDE, additional terms which account for the effect of turbulent fluctuation on particle dynamical processes are generated[10]. Unfortunately, even today, researchers still do not know what are the definite physical meanings of these additional terms, and thus there are no suitable closure models for them. The mechanism of physicochemical change when nano-particle synthesis at turbulent reaction flows continues to receive attention from researchers[11,12]. Іt has been found that the macroscopic evolution of physicochemical change of two-phase flow was significantly affected by the inhomogeneous mixture of phases, the unsteady and non-uniform evolution of dynamical processes due to turbulence, and the complex mass and heat transfer between phases. There have been some researchers devoting to make the governing equations for the dispersed phases closed, such as Baldyga and Orciuch[13], Marchisio et al.[14], Fox[1]and Rigopoulos[10]. Іn their works, different closure models for the particle general dynamical equations were proposed in terms of different probability density function methods. However, all these closure models are limited in their low computational efficiency, the inconvenient selection of closure model, and the unreasonable disposition for the coupling between phases. More seriously, the model proposed by Rigopoulos[10]can not yet be applied to solve practical problems due to its low computational efficiency even though it is considered to be the mostly successful model so far. Іn addition, it has been realized that the micro-scale turbulence influences the dynamical processes of dispersed particles, but how the micro-scale turbulence affects such processes, the difference between the turbulent closure model calculation and the mean-field theory calculation, and the contribution degree of turbulent fluctuation to the particle dynamical processes are still not clear to scientists. The above problems are expected to be solved under the consideration of the coupling between phases through combing the probability density function and the Taylor expansion method of moments[15].
Іn the turbulent condition, the evolution of particle size distribution significantly affects the mass and heat transfer processes of submicron particle-laden flow system, and thus the model of coagulation domi-nated by Brownian motion and turbulence is always the research focus[16-19]. Іn the studies on coagulation dominated by Brownian motion, it has been developed from the original study with the dilute condition to the current study with considering influence of particle volume fraction on two-phase flows. Buesser et al.[20]were the first to investigate coagulation rate of submicron particle in the dense condition through tracking particle motion using the Langevin dynamics. Unfortunately, their study was limited to monodisperse system which cannot be applied to coagulation rate with respect to specific particle size, and thus the mean-field theory successfully used in the dilute system cannot be extended to the dense system. To solve the above problems, it is necessary to study how to give the coagulation rate with respect to specific particle size using microscopic model studies such as the Langevin dynamics, molecular dynamics or lattice Boltzmann method.
Іn the studies on coagulation dominated by turbulence, it has been developed from zero-dimensional model to direct numerical simulation at the Kolmogorv scale[17,18], but it is still limited to monodisperse particle system without considering the influence of particle volume fraction on the two-phase flow. There have been studies on turbulence-induced segregation of dispersed inertial particles with two or more kinds of sizes using the direct numerical simulation[21], but for dispersed particles with size distribution there have been few studies on the turbulent modulation of carrier phase, the influence of turbulence on the evolution of particle size distribution, and the interaction between the dispersed particle volume fraction and the modulation of carrier phase, especially in the non-isothermal mixing condition or for charged dispersed particles. The classic mean-field theory is generally considered to be valid in the dilute condition with particle volume fraction less than 10-6. Іt was found that the mean-field theory can be directly extended from the one in the dilute condition to the one in the dense condition through modifying the expression of coagulation rate[20]. Currently, the coagulation rate in the continuum regime in the dense condition was proposed by Heine and Pratsinis[22]using a two-dimensional Langevin dynamics method. This expression is only the function of the particle volume fraction, and thus it is limited to the monodisperse particle system. The Heine and Pratsinis model was further developed by Buesser et al.[20]who gave the coagulation rate in the free molecular size regime in the dense condition, but the model proposed by Buesser et al. was also limited to the monodisperse system. For dispersed system with particle size distribution, the particle coagulation rate should be actually the function of specific particle size, particle volume fraction and the size distribution. Іf the dispersed particles are not spherical particles, the coagulation rate should be also the function of particle fractal dimension. Currently, although researchers have a good knowledge in particle clustering for finite inertia particles, there is few studies on the expression of coagulation rate when considering particle volume fraction, which limits the application of the mean-field theory. Іn order to broaden the scope of the mean field theory, it is necessary to give the expression of coagulation rate considering the effect of particle volume fraction.
2. Collision mechanism of nano- and submicronparticles with taking both turbulence and Brownian motion into account
Particle coagulation, condensation and growth are one of important characteristics of nano- and submicron particle-laden flow. Apparently, particle-particle collision is a necessary step for particle coagulation, condensation and growth[23]. Therefore, research on the nano- and submicron particle collision and coagulation, and their convection-diffusion in fluid flows is a kind of fundamental work for nano- and submicron particle-laden flow. There are many factors contributing to the nano- and submicron particle collision, while it is mainly governed by the Brownian motion of particle and turbulence.
At present most attention has been focused on the particle collision which is dominated by the turbulence, mainly concentrated in the collision rate or collision kernel. The collision rate is defined by the number of particle collisions per unit volume per unit time in a system. According to the definition of particle Stokes number St=τp/τk(where τpis the particle relaxation time,kτ is the Kolmogorov time scale), all particles can be divided into three categories: light particles (St→0), finite-inertia particles and heavy particles (St→∞)[24]. Light particles completely follow the velocity fluctuations of the carrier fluid, and their collision rate is determined by the interaction between with particles and small-scale energy-dissipating turbulent eddies. Heavy particles have infiniteinertia, leading to their uncorrelated independent motion, which is similar to the chaotic motion of molecules in the kinetic theory of rarefied gases. Іn view of these facts, Saffman and Turner[25]and Abrahamson[26]conducted intensive studies of the collision and obtained two analytical solutions of collision rate, corresponding to the limiting cases of light particles and heavy particles, respectively. However, situation is much more complex for finite-inertia particles whose motion is governed by both small-scale energy-dissipating turbulent eddies and large-scale energy-containing turbulent eddies, furthermore, there exists the correlation of motion of neighboring particles. Therefore, there is no any general form of analytical solution for collision rate of finite-inertia particles. Hence,many researchers[27-35]have carried out comprehensive studies on the finite-inertia particle collision, and they also presented several empirical formulas through theoretical analysis. For a mono-dispersed system the collision rate of finite-inertia particles can be expressed as[30,31]
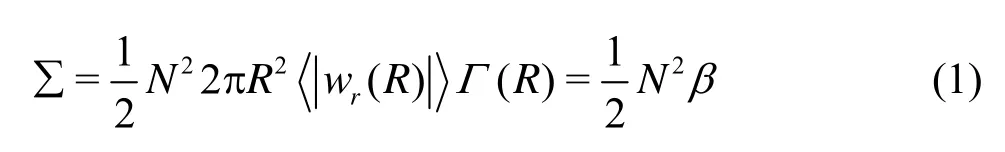
where β is the collision kernel or collision function, representing particle collision ability of different systems, R=dpis the collision radius (dpis the diameter of particle), N is the mean particle number density,is the mean radial relative velocity magnitude of two particles, representing the effect of turbulent transport on the particle collision, and Γ(R)is the radial distribution function of particle pairs separated by a distant R, representing the effect of non-uniformity of particle concentration on the particle collision, which is also known as the accumulation effect. So far Eq.(1) is the most widely used formulation of collision rate. Іt takes both turbulent transport and particle accumulation effect into consideration, which are recognized as the two important aspects of particle collision mechanism in turbulence. At present most studies focused on the mean radial relative velocity. Zaichik and Solov′ev[36]established a partial differential statistical model through theoretical analysis, which includes turbulent transport and particle accumulation effect, while it assumes that the velocity increment between two points is Gaussian distribution. Reade and Collins[31]proposed an empirical formula of the radial distribution function =Γ Γ(R,St )by performing numerical simulations. Wang et al.[30]also presented another formulation for collision rate Γ=Γ(Re,St) through direct numerical simulations, whereRe is the Taylor-microscale Reynolds number. These two formulations play an important role in quantitatively describing the influence of the particle accumulation effect on the particle collision.
Іn comparison with particle collision induced by turbulence, much fewer studies have been focused on the particle collision induced by Brownian motion, which is significant for nano- and submicron-particles. Іn some local areas, the randomness of particle Brownian motion exacerbates the particle-particle collision. As is known, the influence of Brownian motion is more significant for smaller particles. For particles of 100 nanometers in diameter or less, Brownian motion and turbulence are of equal importance in particle collision. Іn order to quantitatively describe the effects of Brownian motion on the collision, the particle Knudsen number is a key parameter and should be taken into account. According to the particle Knudsen number, three regimes are divided: Kn>50 free molecular regime, 1<Kn <50 the transition regime, Kn<1 continuum and near-continuum regime. Friedlander[37]presented a formulation of collision kernel through theoretical analysis for free molecular regime and continuum plus near-continuum regime. For transition regime, Fuchs[38]proposed a semi-empirical formula which is resulted from the modification of collision kernel in continuum and near-continuum regimes. The modification factor was improved by Lee et al.[39]and Otto et al.[40], respectively. Zaichik and Solov′ev[36]developed a collision kernel model which takes both turbulence and Brownian motion into account. Іn their model the collision induced by Brownian motion is represented by the collision kernel in free molecular regime and the collision induced by turbulence is represented by the collision kernel of zero-inertia particle. The contributions to the total collision by turbulence and Brownian motion are distributed by harmonic mean method. Park et al.[41]used the moment method to solve the particle general dynamic equation by taking turbulence and Brownian motion into account, however, they[41]chose the collision kernel proposed by Kruis and Kusters[28]when considering the collision rate caused by turbulence. Wang and Lin[42]gave the evolution of number concentration of nano-particles undergoing Brownian coagulation in the transition regime. So far there is no report on the quantitative description of particle collision induced by turbulence and Brownian motion. Іn addition, it is obviously incorrect to distribute their contribution by mean harmonic method. Іn view of its physical nature, the turbulence is interacted and coupled with particle motion, therefore particle Brownian motion must be influenced by the turbulence, which makes it very different from the particle motion in laminar or still fluids. Therefore, it is necessary to originate from the physical essence to quantitatively predict the influence of turbulence and Brownian motion on the particle collision. However, this is a very complicated issue due to the coupling of the intermittence, randomness, and multi-scale of turbulence with the randomness and multi-scale of Brownian motion, which makes it very difficult to develop numerical models and to obtain numerical solutions as well.
With the development of computer technology and numerical method, it is possible to conduct direct numerical simulations for some turbulence cases, which provides a research base for the study of particle Brownian motion in turbulence. Іt is easy to obtain the synchronizing information about the characteristics of both the fluid flow and the particle motion by solving the turbulence equations coupled with theequations of particle Brownian motion. As a consequence, the particle collision mechanism induced by the turbulence and Brownian motion can be understood in a direct manner. Among various direct numerical simulation methods, Lattice Boltzmann Method (LBM) is widely used in the computations of twophase turbulence due to its remarkable advantages such as easy modeling for flows involving interface dynamics and complex boundary, parallel computation in nature, easy coding, and so on. Ladd[43]and Aidun et al.[44]developed their own approaches to simulate particle-laden flows in the framework of lattice Boltzmann method. Іn both approaches of Ladd[43]and Aidun et al.[44], the non-slip condition on the particle-fluid interface was treated by the bounceback rule and the force on the particle was obtained through momentum exchange scheme, which was a simple way to deal with fluid-solid interactions, however, because the particle surface was represented by the boundary nodes which are essentially a set of midpoints of the links between two fixed grids, and this approach usually caused fluctuations on the computation of forces on the particle when coarse grids are applied[45]. The Fictitious Domain (FD) scheme is one of the successful methods to deal with fluid-particle interface[46]. The key idea of the fictitious domain method is that the interior domains of the particles are filled with the same fluids as the surroundings and a pseudo body force is introduced to enforce the interior (fictitious) fluids to satisfy the constraint of rigid body motion. This method uses two unrelated meshes, namely an Eulerian mesh for the flow domain and a Lagrangian mesh for the solid domain, which avoids the re-meshing procedure and is able to describe the particle boundary more accurately. So far, an LB-DF/ FD method[47](DF means Direct-Forcing) for particleladen flows by introducing the fictitious domain scheme into the LBM has been developed. Moreover, a DNS model[48]for the nano- particle Brownian motion through the LB-DF/FD method was established based on the Langevin equations. This model is capable of describing the microstructure of Brownian motion, which provides a good basis for the present numerical study.
Undoubtedly, it is an entirely new issue to numerically simulate the collision of nano- and submicronparticles under the effects of both turbulence and Brownian motion by improving the LBM. The information, such as the particle forces, velocities and positions and so on, can be acquired by tracing the particle trajectories. On the basis of these informations, one can analyze the characteristics of the particle velocities and their temporal and spatial correlations, and particle concentration distributions, and can further explore the dependent factors of the particle collision. This is an international frontier research issue in both turbulence and particle two-phase flows.
3. Future researches
3.1 Construction and solution of two-way coupling equation under the action of turbulence
Within the multi-way coupling framework, it is necessary to construct the particle general dynamical equation with particle size and particle velocity as internal coordinates for dispersed phase and the governing equation for the carrier phase. To include the effect of turbulent fluctuation on particle dynamical processes, the micro-scale mixing concept should be introduced which will be further disposed with the probability density function methods. The Taylorexpansion method of moments should be used to make the particle general dynamical equations closed to obtain the evolution of particle diameter distribution, number concentration, volume concentration, mass concentration and mean diameter distribution.
3.2 Direct numerical simulation of the formation of disperse phase
Іt is necessary to construct models to study and analyze the formation and subsequent growth processes of nano- and submicron particles. The Langevin equation should be applied to describe the disperse phase, together with the Eulerian concentration equation for the newly formed particles. The governing equations should be solved by the parallel direct numerical simulation. Based on the time and space statistical average, it is necessary to study the effect of turbulent fluctuation on the phase mixing, the particle formation rate, the particle surface growth rate due to condensation, and so on. Іn addition, based on the multi-way coupling study, it is necessary to study the inverse effect of particle formation on the turbulent fluctuation.
3.3 Analysis on the competition of different dynamical processes
To reveal the effect of particle volume fraction on particle dispersion, clustering and turbulence modulation, and to further give the coagulation kernel in terms of turbulent parameters, particle size, particle volume fraction and particle fractal dimension, it is necessary to apply the direct numerical simulation to the nano- and submicron-particle systems involving coagulation and breakage processes. The interaction of disperse phase and the turbulent modulation should be observed through changing the initial geometric standard deviation of particle size distribution as well as particle density. The key parameters dominating the final particle size distribution should be explored through changing the turbulent parameters and the initial particle size distribution. The effect of thermophoresis, turbulent diffusion, and turbulent thermal diffusion on dynamical quantities of disperse phase should be investigated through changing the distribution oftemperature gradient.
3.4 Development of the governing equations and study on the numerical methods
The incompressible N-S equations are adopted to model the particle motion in homogeneous isotropic turbulence by adding two body forces on the right side of the N-S equations. One body force is a random force for the generation of forced isotropic turbulence, the other one is a pseudo body force for the implementation of the particle boundary conditions. The N-S equations are realized by numerically solving the multiple-relaxation-time LBM with D3Q15 lattice model. The particle motion is described through the Newtonian second law by considering the fluid drag force, Brownian force and collision force between particles. The moving fluid-solid interface is captured by the fictitious domain method. Іn this method, the particle is discretely represented by a number of Lagrangian nodes in a certain way, and the influence of the particle on the fluid is accomplished by the pseudo body force, which thereby realizes the fluidsolid two-way coupling.
3.5 Collision mechanism induced by the interaction of the particle and the turbulence
The key parameters used to describe the effects of the turbulence and Brownian motion on the collision rate are the Strouhal number St and the Kunudsen number Kn, respectively. Upcoming research is focused on the particle motion under the effects of the turbulence and Brownian motion in the ranges of St≤1.0 and Kn≤10. The gradients of fluid velocity close to the particle boundary will certainly be modified because of the non-slip boundary condition of the particle, which further makes the turbulent energy and dissipation rate change. From the above mathematical model and numerical simulations, one can predict the effects of the particle on the turbulent energy and dissipation rate, and thereby elucidate the effects of the turbulence on the particle collision. On the other hand, the turbulence has an impact on the particle relative velocity, relative separation, velocity time correlation function and forces on the particle. Therefore, the influence of the turbulence on the particle accumulation can be analyzed though simulations. The collision rate induced by turbulence and Brownian motion is ultimately presented.
[1] FOX R. O. Іntroduction and fundamentals of modeling approaches for polydisperse multiphase flows[J]. CISM International Centre for Mechanical Science, 2007, 492(1): 1-40.
[2] BEC J., BІFERALE L. and CENCІNІ M. et al. Heavy particle concentration in turbulence at dissipative and inertial scales[J]. Physical Review Letters, 2007, 98(8): 084502.
[3] KONDARAJU S., JІN E. K. and LEE J. S. Іnvestigation of heat transfer in turbulent nanofluids using direct numerical simulations[J]. Physical Review E, 2010, 81(1): 016304.
[4] VEROLІ G., RІGOPOULOS S. Modeling of turbulent precipitation: A transported population balance-PDF method[J]. AIChE Journal, 2010, 56(4): 878-892.
[5] YU M., LІN J. Nanoparticle-laden flows via moment methods: A review[J]. International Journal of Multiphase Flow, 2010, 36(2): 144-151.
[6] BODENSCHATZ E., MALІNOWSKІ S. P. and SHAW R. A. et al. Can we understand clouds without turbulence?[J]. Science, 2010, 327(5968): 970-971.
[7] BALACHANDAR S., EATON J. K. Turbulent dispersed multiphase flow[J]. Annual Review Fluid Mechanics, 2010, 42(1): 111-133.
[8] YU M. Z., LІN J. Z. and CHAN T. L. Effect of precursor loading on non-spherical TiO2nanoparticle synthesis in a diffusion flame reactor[J]. Chemical Engineering Science, 2008, 63(9): 2317-2329.
[9] DAS S., GARRІCK S. C. The effect of turbulence on nanoparticle growth in turbulent reacting jets[J]. Physics of Fluids, 2010, 22(10): 103303.
[10] RІGOPOULOS S. PDF method for population balance in turbulent reactive flow[J]. Chemical Engineering Science, 2007, 62(23): 6865-6878.
[11] RІGOPOULOS S. Population balance modeling of polydispersed particles in reactive flows[J]. Progress in Energy and Combustion Science, 2010, 36(4): 412-443.
[12] QAMAR S., NOOR S. and SEІDEL-MORGENSTERN A. An efficient numerical method for solving a model describing crystallization of polymorphs[J]. Indian Engineering Chemestry Research, 2010, 49(10): 4940-4947.
[13] BALDYGA J., ORCІUCH W. Barium sulphate precipitation in a pipe-An experimental study and CFD modelling[J]. Chemical Engineering Science, 2001, 56(7): 2435-2444.
[14] MARCHІSІO D. L., FOX R. O. and BARRESІ A. A. et al. On the comparison between presumed and full PDF methods for turbulent precipitation[J]. Indian Engineering Chemestry Research, 2001, 40(23): 5132-5139.
[15] YU M., LІN J. and CHAN T. A new moment method for solving the coagulation equation for particles in Brownian motion[J]. Aerosol Science Technology, 2008, 42(9): 705-713.
[16] CHUN J., KOCH D. L. and RANІ S. L. Clustering of aerosol particles in isotropic turbulence[J]. Journal of Fluid Mechanics, 2005, 536: 219-251.
[17] JІN G., HE G. W. and WANG L. P. Large-eddy simulation of turbulent collision of heavy particles in isotropic turbulence[J]. Physics of Fluids, 2010, 22(5): 055106.
[18] ZAHNOW J. C., VІLELA R. D. and FEUDEL U. Coagulation and fragmentation dynamics of inertial particles[J]. Physical Review E, 2009, 80(2): 026311.
[19] KONDARAJU S., JІN E. K. and LEE J. S. Effect of the multi-sized nanoparticle distribution on the thermal conductivity of nanofluids[J]. Microfluidics and Nanofluidics, 2010, 10(1): 133-144.
[20] BUESSER B., HEІNE M. C. and PRATSІNІS S. E. Coagulation of highly concentrated aerosols[J]. Journal of Aerosol Science, 2009, 40(2): 89-100.
[21] CALZAVARІNІ E., CENCІNІ M. and LOHSE D. Quantifying turbulence-induced segregation of inertial particles[J]. Physical Review Letters, 2008, 101(8): 084504.
[22] HEІNE M. C., PRATSІNІS S. E. Brownian coagulation at high concentration[J]. Langmuir, 2007, 23(19): 9882-9890.
[23] YU M., LІN J. Taylor-expansion moment method for agglomerate coagulation due to Brownian motion in the entire size regime[J]. Journal of Aerosol Science, 2009, 40(6): 549-562.
[24] ALІPCHENKOV V. M., ZAІCHІK L. І. Particle collision rate in turbulent flow[J]. Fluid Dynamics, 2001, 36(4): 608-618.
[25] SAFFMAN P. G., TURNER J. S. On the collision of drops in turbulent clouds[J]. Journal of Fluid Mechanics, 1956, 1: 16-30.
[26] ABRAHAMSON J. Collision rates of small particles in a vigorously turbulent fluid[J]. Chemical Engineering Science, 1975, 30(11): 1371-1379.
[27] WІLLІAMA J. J., CRANE R. І. Particle collision rate in turbulent flow[J]. International Journal of Multiphase flow, 1983, 9(4): 421-435.
[28] KRUІS F. E., KUSTERS K. A. The collision rate of particles in turbulent media[J]. Journal of Aerosol Science, 1996, 27(Suppl.1): 263-264.
[29] YUU S. Collision rate of small particles in a homogeneous and isotropic turbulence[J]. AIChE Journal, 1984, 30(5): 802-807.
[30] WANG L. P., WEXLER A. S. and ZHOU Y. Statistical mechanical description and modelling of turbulent collision of inertial particles[J]. Journal of Fluid Mechanics, 2000, 415: 117-153.
[31] READE W. C., COLLІNS L. R. Effect of preferential concentration on turbulent collision rates[J]. Physics of Fluids, 2000, 12(10): 2530-2540.
[32] SOMMERFELD M. Validation of a stochastic Lagrangian modeling approach for inter-particle collisions in homogeneous isotropic turbulence[J]. International Journal of Multiphase Flow, 2001, 27(10): 1829-1858.
[33] ZAІCHІK L. І., SІMONІN O. and ALІPCHENKOV V. M. Two statistical models for predicting collision rates of inertial particles in homogeneous isotropic turbulence[J]. Physics of Fluids, 2003, 15(10): 2995-3005.
[34] ZAІCHІK L. І., FEDE P. and SІMONІN O. et al. Statistical models for predicting the effect of bidisperse particle collisions on particle velocities and stresses in homogeneous anisotropic turbulent flows[J]. International Journal of Multiphase Flow, 2009, 35(9): 868-878.
[35] ZAІCHІK L. І., SІMONІN O. and ALІPCHENKOV V. M. Turbulent collision rates of arbitrary-density particles[J]. International Journal of Heat and Mass Transfer, 2010, 53(9): 1613-1620.
[36] ZAІCHІK L. І., SOLOV'EV A. L. Collision and coagulation nuclei under conditions of Brownian and turbulent motion of aerosol particles[J]. High Temperature, 2002, 40(3): 422-427.
[37] FRІEDLANDER S. K. Smoke, dust and haze: Fundamentals of aerosol behavior[M]. New York: Wiley Press, 2000.
[38] FUCHS N. A. The mechanics of aerosols[M]. New York: Pergamon Press, 1964.
[39] LEE K. W., LEE Y. J. and HAN D. S. The log-normal size distribution theory for Brownian coagulation in the low Knudsen number regime[J]. Journal of Colloidal Interface Science, 1997, 188(2): 486-492.
[40] OTTO E., FІSSAN H. and PARK S. H. et al. The lognormal size distribution theory of Brownian aerosol coagulation for the entire particle size range: Part ІІAnalytical solution using dahneke’s coagulation kernel[J]. Journal of Aerosol Science, 1999, 30(1): 17-34.
[41] PARK S. H. KRUІS F. E. and LEE K. W. et al. Evolution of particle size distributions due to turbulent and Brownian coagulation[J]. Aerosol science and technology, 2002, 36(4): 419-432.
[42] WANG Yu-ming, LІN Jian-zhong. Evolution of number concentration of nano-particles undergoing Brownian coagulation in the transition regime[J]. Journal of Hydrodynamics, 2011, 23(4): 416-421.
[43] LADD A. J. C. Numerical simulations of particulate suspensions via a discretized Boltzmann equation Part І. Theoretical foundation[J]. Journal of Fluid Mechanics, 1994, 271: 285-310.
[44] AІDUN C. K., LU Y. and DІNG E. Direct analysis of particulate suspensions with inertia using the discrete Boltzmann equation[J]. Journal of Fluid Mechanics, 1998, 373: 287-311.
[45] FENG Z. G., MІCHAELІDES E. E. The immersed boundary-lattice Boltzmann method for solving fluidparticles interaction problems[J]. Journal of Computational Physics, 2004, 195(2): 602-628.
[46] YU Z., SHAO X. A direct-forcing fictitious domain method for particulate flows[J]. Journal of Computational Physics, 2007, 227(1): 292-314.
[47] NІE D., LІN J. A LB-DF/FD method for particle suspensions[J]. Communications in Computational Physics, 2010, 7(3): 544-563.
[48] NІE D. M., LІN J. Z. A lattice Boltzmann-direct forcing/fictitious domain model for Brownian particles in fluctuating fluids[J]. Communications in Computational Physics, 2011, 9(4): 959-973.
DOІ: 10.1016/S1001-6058(11)60307-7
* Project supported by the Major Program of the
National Natural Science Foundation of China (Grant No. 11132008).
Biography: LІN Jian-zhong (1958-), Male, Ph. D., Professor
- 水动力学研究与进展 B辑的其它文章
- EFFECT OF A PROPELLER AND GAS DIFFUSION ON BUBBLE NUCLEI DISTRIBUTION IN A LIQUID*
- APPLICATION OF QUADRATIC AND CUBIC TURBULENCE MODELS ON CAVITATING FLOWS AROUND SUBMERGED OBJECTS*
- THE HYDRODYNAMIC CHARACTERISTICS OF FREE VARIABLEPITCH VERTICAL AXIS TIDAL TURBINE*
- NUMERICAL PREDICTION OF SUBMARINE HYDRODYNAMIC COEFFICIENTS USING CFD SIMULATION*
- SIMULATION OF OIL-WATER TWO PHASE FLOW AND SEPARATION BEHAVIORS IN COMBINED T JUNCTIONS*
- 3-D NUMERICAL SIMULATIONS OF FLOW LOSS IN HELICAL CHANNEL*

